Article
citation information:
Chernets, M., Wrona, R., Chernets,
Y. Analytical study of durability and contact characteristics of metal-polymer
composite sliding bearings for means of transport. Scientific
Journal of Silesian University of Technology. Series Transport. 2024, 123, 5-21.
ISSN: 0209-3324. DOI: https://doi.org/10.20858/sjsutst.2024.123.1.
Myron
CHERNETS[1], Rafał WRONA[2], Yuriy CHERNETS[3]
ANALYTICAL
STUDY OF DURABILITY AND CONTACT CHARACTERISTICS OF METAL-POLYMER COMPOSITE
SLIDING BEARINGS FOR MEANS OF TRANSPORT
Summary. The field of
application of metal-polymer bearings is vast: transport of various types,
processing industry equipment, medical equipment, various types of maintenance
equipment, etc. With the use of the developed generalized author's analytical
method of metal-polymer plain bearings on the basis of which it is laid the
author's research methodology of materials wear kinetics at sliding friction
(dry, lubricated), calculation of their durability is carried out. Contact
parameters are also determined. Metal-polymer bearings with a bushing made of
PA6, PA66 polyamide and PA6 based composites filled with glass (PA6 + 30GF) and
carbon (PA6 + 30CF) dispersed fibres, molybdenum disulphide (PA6 + MoS2)
and oil filled cast polyamide (PA6 + oil) are considered. These polyamides
(unfilled and filled), as self-lubricating materials, are widely used in this
type of dry friction sliding bearings. The predictive estimation of durability
of investigated bearings depending on loading, polymer materials Young's
modulus, their wear resistance and sliding friction coefficient is executed.
Regularities of change from the specified factors of bearing's durability and
the maximum contact pressures are established. Experimental indicators,
diagrams, and wear resistance characteristics of the specified polymeric
materials are presented. The results of researches of sliding friction
coefficient and modulus of elasticity for carbon steel (0.45%C) – polyamides
tribocouples are presented.
Keywords: metal-polymer sliding bearings, analytical
method, polyamides, PA6, PA66, composites PA6 + 30GF, PA6 + 30CF, PA6 + MoS2,
PA6 + oil, Young's modulus, wear resistance, coefficient of friction,
durability, contact parameter
1. INTRODUCTION
Metal-polymer plain
bearings (MP) are used in a variety of machines, equipments, and devices in
automotive, mechanical engineering, instrumentation, aerospace, rocket and
space technologies, etc.; in all types of processing industry – food, textile,
pharmaceuticals, chemical, etc. They are commonly found in household
appliances, computer, office, medical, measuring equipment, and other related fields.
They operate in water, gas, liquid, boundary and dry friction. The
self-lubricating MP bearings offer numerous advantages in comparison to rolling
bearings, including simplicity or lack of maintenance, high manufacturability,
a wide range of shaft diameters (ranging from micro to large), small
cross-sectional dimensions, significant shaft speed, ability to operate under
dynamic and shock loads, high damping capacity, low noise generation, and the
ability to operate in polluted technological environments or water, at elevated
and lowered temperatures, in vacuum, and other characteristics.
The reliability of
MP bearings is particularly important because many technical devices are forced
to operate without the use of lubricants due to various reasons and production
conditions. In fact, such conditions may be provided for in the operation
of different types of vehicles or may arise under different circumstances.
Given that dry friction
causes significantly more intense wear compared to the boundary friction, for
MP bearings it is very relevant and necessary to calculate their durability and
load carrying capacity in the design. However, the corresponding effective
methods of calculation of MP bearings in the literature are virtually absent.
Developed calculation [1-8] or numerical methods [9-11] of the study of metal
plain bearings have not found practical application for calculating the
durability and load carrying capacity of MP bearings. In the existing studies
of MP sliding bearings [12-14], the authors use the well-known linear Archard`s
law of abrasion / adhesive wear. However, even in MP dry friction bearings, not
to mention metal bearings, where dry friction is almost non-existent, the
mechanism of abrasive wear is unlikely. Regarding the presence of a dominant
mechanism of adhesive wear in metal-polymer couples, [15] states that the wear
of polymers at sliding friction is a combination of three mechanisms (fatigue,
adhesive, and sometimes abrasive). Which of them will be the main and which are
concomitant depends on the polymer properties, the friction conditions and the
course of wear. Under certain conditions, the polymeric material is transferred
to the metal counterpart, forming a thin adhesive film, which is a sign of
adhesive wear as the main process [16-18]. However, in [16] it is noted that at
friction coefficients greater than 0.3, characteristic of MP dry friction
pairs, surface fracture (wear) of polymers occurs by the mechanism of surface
fatigue under the influence of shear stresses (specific friction forces).
Adhesion wear can also partially occur as a minor concomitant process.
Considering this, the author's methodology for studying the materials wear
kinetics during sliding friction [19-22] and, accordingly, the calculation
method for studying the wear of metal [21-25] and MP bearings [26, 27] assumes
a fatigue mechanism, and takes into account changes in conditions of contact
interaction due to wear.
MP bearing bushings are made of various
polymers (polyamides – PA, polyacetal – POM, polytetrafluoroethylene – PTFE,
polyester ketones – PEEK, ultra-high molecular weight polyethylene – UHMWPE,
etc.) and filled composites based on them [11]. Materials (glass and carbon
fibres, molybdenum disulphide, graphite, bronze powder, polytetrafluoroethylene,
etc.) with different volumetric contents are used as fillers in the polymer
matrix, the function of which is increasing the wear resistance and durability
of bearings. In particular, a widely used polymer for MP bearings is polyamide
PA66 and partially PA6. Polyamide based composite materials are much more
widely used, in particular PA6 filled with fibreglass (PA6 + 30GF), carbon
fibre (PA6 + 30CF), molybdenum disulphide (PA6 + MoS2), oil filled
cast polyamide (PA6 + oil).
The literature presents
a number of results of tribological experimental studies of different polymeric
materials used in MP plain bearings [28-36]. In particular, in [28] the wear
and friction coefficient of PA6 based polyamide composites coupled with AISI 02
steel (0.90% C, 1.6% Mn) were studied according to the pin-on-disk scheme
during dry friction. In [29], the tribological behaviour of various bearings
with polymer bushings coupled with steel shaft during dry friction was studied:
polyacetal (POM-Delrin), cast polyamide PA6, and others. The work [30] is
devoted to the study and evaluation of wear resistance of bearings based on PE
(polyethylene), PA, POM, PTFE and Bakelite. Study [31] establishes the effect
of sliding speeds on friction and wear in bearings made of composites PA66,
PA66 + 18PTFE and PA66 + 20GF + 25PTFE at room temperature. Tribological
analysis of the dry friction behaviour of cast nylon (PA6) for the connecting
rod plain bearing was performed in [32]. G. Kalácska presents in [33] the
results of pin-on-disk wear testing of twenty-one engineering polymers, both
basic and composites. In [34], the patterns of friction and wear of PA6 and ABS
(acrylonitrile butadiene styrene) sliding on metal under dry friction
conditions were studied on a pin-on-disc tribometer. [35] is devoted to the
study of the tribological properties of PA6 polyamide in dry friction at
different speeds and loads. In [36], the coefficient of friction, friction
force and temperature of six polymer composites were investigated according to
the ball-on-disk scheme under dry friction: cast polyamide PA6G + oil, PA6G +
MoS2, polyacetal POM + Al, polyterephthalate ethylene PET + PTFE,
PTFE + Bronze, PTFE + Graphite. Although the results of these studies are quite
extensive, it is not possible to establish the wear resistance characteristics
of polymers in order to calculate the durability of plain bearings.
The presented article is
the result of the investigation conducted by the author's calculation method
for metal-polymeric plain bearings.
- durability of the MP
bearing at allowable wear of the polymeric bushing;
- initial maximum
contact pressures;
- initial contact angle;
- wear resistance of
basic polyamides PA6, PA66;
- wear resistance of
filled composites РА6 + 30GF, РА6 + 30CF, РА6 + MoS2, РА6 + oil;
- influence of fillers
on the wear resistance of the studied polymeric materials;
- effect of contact
pressure on the coefficient of sliding friction.
2. METHODS OF
CALCULATION OF METAL-POLYMER BEARINGS
The plane contact problem of the linear
theory of elasticity is studied. In the plain bearing (Fig. 1) the shaft 2,
which is under the action of the reduced force 
rotates
at angular velocity
=
const. Under the influence of full external static load 
, the shaft journal 1 and the bushing 2
located in the housing 3 are contacted in the area 
on which act unknown in magnitude contact
pressures
. A radial clearance 
will be guaranteed between the outer radius of
the steel shaft 
and
the inner radius of the polymer bushing
. The elements materials of MP bearing
have significantly different strength, elastic characteristics, wear resistance
(strength characteristics of 8… 10 times, Young's modulus of 50… 200 times,
wear resistance by 2… 3 orders of magnitude). This fundamentally affects their
contact strength, bearing capacity, contact parameters and, of course,
recourse. When rotating, the shaft 2 wears along the contour 
and the bushing 1 - in the area 
Fig. 1. Metal-polymer plain
bearing: general view and scheme
According to the
tribokinetic mathematical model of wear at sliding friction [20, 21] and the
method of calculation of plain bearings [22-25] for the forecast estimation of
durability at the given bushing wear
the following expression is used
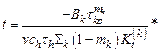
[20, 21]:
(1)
where
- wear resistance characteristics
of tribocouple materials, which are established by the results of model
triboexperimental studies [20, 21]; k
is shaft or bushing index (Fig. 1); 
- sliding speed; ch is the wear rate
coefficient; 
- respectively, the coefficients of mutual overlap of the bushing and
the shaft; 
, 
- relative wear in the
tribosystem.
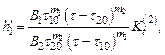
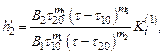(2)
The specific force of
friction is determined by the Amontons-Coulomb law.
(3)
where 
is the coefficient
of sliding friction.
The initial maximum contact pressure 
will occur along
the line of force 
and is determined
by the formula [20, 21]:
(4)
where
– Young's moduli
and Poisson's ratios of the materials of the shaft 1 and bushing 2.
As a result of wear of the composite bushing,
the initial maximum contact pressures will decrease, i.e. in the tribocontact there
will be wear contact pressures
, which are
determined as follows:
(5)
where 
– change in initial pressures due to wear.
To find them, we used the dependence given in
[20, 21]:
(6)
where 
; 
>0 – wear rate indicator; 
The initial specific friction force during the polymer
bushing wear will decrease.
(7)
where 
is the unknown contact semiangle occurring due
to bushing wear.
In the studied type of contact problem, the
initial contact angle 
is also unknown.
Determination of the initial contact semiangle 
is carried out
under the equilibrium condition of forces acting on the shaft 2.
(8)
Taking into account the
expression for the coefficient
, it is expressed as:
(9)
To determine the
tribocontact semiangle 
, which outlines the contact area during wear, the following condition
is used:
(10)
3. MATERIALS, TRIBOEXPERIMENT
To calculate the MP bearing durability according
to (1) should be determined the wear resistance characteristics of materials in
tribocouple steel-polymer. The author's method of model research of materials
wear [20, 21] involves several levels of contact pressure in the tribosystem
and provides determination at the same time both wear (mass or linear) of
samples, and specific force of friction
Model triboexperiments are performed according
to the pin-on-disk friction scheme, which ensures the constancy of the initial
external conditions during the study, namely the contact pressures and sliding
speed, the contact temperature of the experimental tribocouple. This approach
to triboexperimental research significantly expands the information on the wear
resistance of materials in a certain range of contact pressures, in contrast to
the standards ISO 7148-2 and ASTM G99, which provides to conduct experimental
studies at one value of contact pressure. In the author's methodology of
studying the material’s wear kinetics, it is accepted that the level of 
unambiguously
determines their wear rate. It should be noted that it is the coefficient of
sliding friction f determines the
level of t in the tribocontact. That is, at the same level of contact pressure, its value can
vary several times depending on the type of friction (dry, semi-dry friction,
boundary).
The material’s wear resistance characteristics in the tribocouple
are determined by the results of model triboexperimental studies as invariant
wear resistance parameters in the selected range . In the
following, they are necessary to calculate the durability or wear of plain
bearings by the above method.
According to the method of model
triboexperiments, the calculation of experimental indicators of wear resistance
(wear resistance functions) Фі of polymeric materials for each level of
contact pressure pі and correspondingly specific friction force was carried out by using the formula:
Фі=Lі/hі (11)
where L is the path of friction, hi is the linear wear of samples at
the i- th level of pressures pі.
Research program: sliding speed v = 0.4 m/s; contact pressure 
= 2, 4, 6, 8 MPa;
friction path L = 5000… 10000 m;
sample diameter d = 3 mm. Forced
cooling of the friction unit was carried out to ensure the temperature of the
end surface of the polymer sample T =
23 ± 1°C at a relative humidity of 50 ± 5% (standard ISO 7148-2).
For approximation of the wear resistance
experimental indicators Фі the next type of ratio was used [20, 21]:
(12)
The wear resistance characteristics of
tribocouple materials are determined by using the method of the least squares,
where 
is the limiting value of the specific friction
force at which wear at the macro level will be virtually absent.
According to the wear resistance characteristics
determined in this way, graphical indicators (diagrams) of wear resistance are
plotted for each polymer (Fig. 2).
4. RESULTS, DISCUSSION
The source data for calculation of durability
and parameters of contact at dry friction: 
= 5000, 3000 N; D2 = 50 mm; 
; 
0.2 mm; = 6.28 rad / sek; h1* = 0.5 mm.
Metal-polymer bearings from the following
materials of the elements are investigated:
Shaft 2 – carbon
steel 0.45%C, normalized, roughness 
= 0.8-1 μm; Е2 = 210 GPa,
ν2 = 0.3; 
= 1013,
= 2, 
= 0.1 MPa;
polymeric bushing 1 (Table 1).
Tab. 1
Parameters
of bushing materials
|
Wear resistance characteristics |
Thermoplastic polymeric materials |
|||||
|
РА6 |
РА66 |
РА6+ 30GF |
PA6+ МоS2 |
PA6+ 30CF |
PA6+oil |
|
|
В1 ∙1010 |
2.26 |
3.37 |
4.12 |
5.58 |
6.53 |
7.03 |
|
m1 |
1.09 |
1.09 |
1.09 |
1.1 |
1.1 |
1.1 |
|
τ10,
MPa |
0.05 |
0.05 |
0.05 |
0.05 |
0.05 |
0.05 |
|
Young's module E1, GPa |
2.0 |
2.3 |
2.7 |
1.66 |
3.3 |
1.96 |
|
Poisson's
ratio ν1 |
0.4 |
0.4 |
0.41 |
0.4 |
0.41 |
0.4 |
Note: for PA6 + 30GF the volume content of filler
(fibreglass) is 30%.
The results of triboexperimental studies and numerical solution are
presented in Fig. 2-9. Fig. 2 presents diagrams of wear resistance of the above
polymeric materials, and Fig. 3 presents their relative wear resistance.
The figure shows the determined experimental
functions of the wear resistance of polyamides Фi with different markers at four values of the
specific force of friction 
in the
tribocontact. Their location along the axis at the same contact pressures depends on
the coefficient of friction in the studied tribocouple. However, the
qualitative patterns of changes in wear resistance in the form of wear
resistance diagrams are practically the same for these polymer materials. These
graphic indicators of wear resistance - wear resistance diagrams of polyamides
are obtained by approximation of Фі according to (12). They make it possible to
conduct a visual comparative assessment of the wear resistance of these
polyamides at a specific value of . From the
analysis of Fig. 2 it is noticeable which of the polyamides has low wear
resistance (PA6), and which has the highest wear resistance (PA6 + oil). It
should be noted that the wear resistance of polyamides depends non-linearly on
the effective specific force of friction.
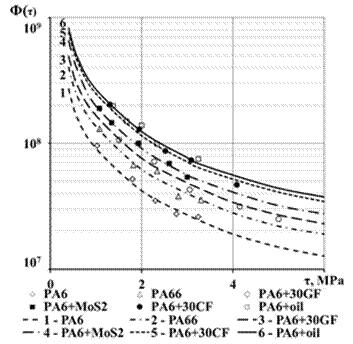
Fig. 2. Diagrams of polyamides wear
resistance
For example, the ranking according to the
criterion of wear resistance of the studied polyamides at 2 MPa relative to the
least resistant polyamide PA6 at 
2 MPa is shown in
Fig. 3.
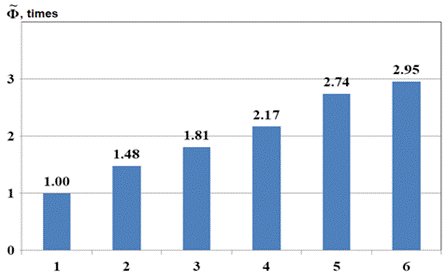
Fig. 3. Relative wear
resistance of polyamides
1 - РА6, 2 - РА66, 3 - РА6 + 30GF, 4 - РА6 + МоS2, 5 - РА6 + 30СF, 6 - РА6 + oil
Since the wear resistance index m (Table 1) of all studied polyamides is
almost the same, in the whole range of changes in their relative wear resistance 
will be almost the same as shown in Fig. 3.
Increasing the durability of polyamides is close to linear.
According to the given data, the calculation of durability of MP bearings
with various materials of bushings (Fig. 4) is carried out.
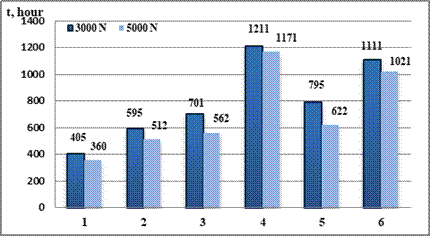
Fig. 4. Durability of MP bearings
There is no increase in the durability in the
order of increasing their wear resistance (Fig. 2) from polymer 1 to polymer 6,
although the wear resistance characteristic B
is increasing (Table 1). This type of sequence does not exist either in
the coefficients of sliding friction (Fig. 5 and 6) or in the value of the
Young's moduli (Table 1, Fig. 9). As a result, the MP bearing with a polymer
sleeve made of a more durable material (PA6 + oil) will not have the highest
durability. The highest durability has a composite PA6 + MoS2. It
depends on the complex influence of these three characteristics. This, in fact,
applies to composites PA6 + 30CF and PA6 + oil.
Fig. 5 shows the relative durability (ranking by durability) of MP bearings
relative to polyamide PA6.
If the durability (Fig. 4) at higher loads is
lower for all materials, then in the case of relative durability (Fig. 5) there
is no such pattern for two polyamide composites - PA6 + MoS2, PA6 +
oil.
As a result of triboexperimental studies, the non-linear nature of the
decrease in the friction coefficient with increasing contact pressure was
established (Fig. 6).
The highest is the coefficient of friction in
the pair РА6 + 30GF - steel, slightly lower - in the pair РА6 + 30СF - steel, and the lowest - in the pair РА6 + МоS2 - steel.
Accordingly, at contact pressures caused by loads = 3000, 5000 N, their values are shown in Fig. 7.
By using the presented method, the maximum
contact pressures р0
(Fig. 8) and contact angles (Fig. 9) were estimated.
The value of the maximum contact pressure р0 under
the same conditions depends only on the value of the modulus of elasticity E of polymeric materials.
As the Young's modulus increases, the rigidity
of the polymer material increases, because of which the contact angle 
decreases and the
maximum contact pressure р0 increases.
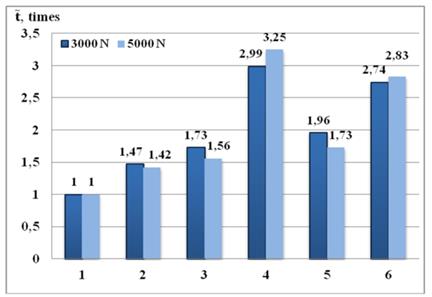
Fig. 5. Relative durability of MP
bearings
It is known that in the design calculation of
metal plain bearings in engineering practice, the conventional method based on
the average pressure p as a criterion for their bearing capacity,
calculated according to a simplified method, is used. It is assumed that 
when the contact angle 
114.6º =
const, i.e.
(13)
where [p] is
the allowable contact pressure for less durable material.
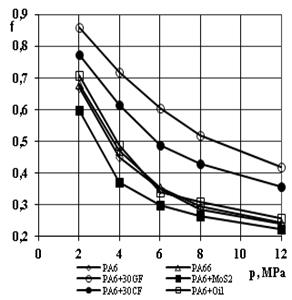
Fig. 6. Experimental
dependence of the average coefficient of sliding friction on the pressure in
polyamide-steel tribocouples
Fig. 7. The effect of load and type
of polyamide on the coefficient of sliding friction
A modified formula for maximum contact pressure рmax is given in
[37], where the cosine law of its distribution by contact area at angle = 180º is
laid down.
(14)
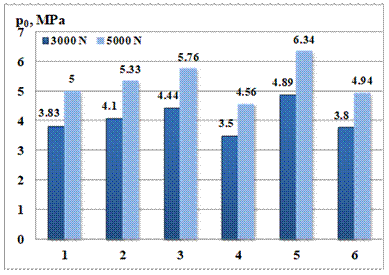
Fig. 8. Maximum contact pressures
in the MP bearing with
various types of polyamide bushings
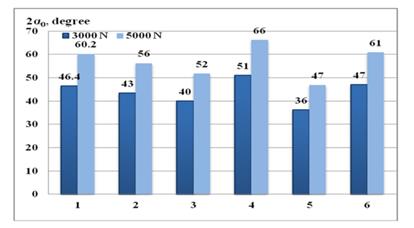
Fig. 9. Contact angles
These formulas do not take into account both the
radial clearance 
and the elastic
characteristics
of the materials of bearing elements.
As was shown above, the characteristics of the
bearing and materials have a decisive influence on the contact parameters - р0, . The specified
contact conditions of the bearing elements in these methods are inappropriate.
There is always a certain radial clearance here, which ensures reliable
operation of the bearing. It should also be noted that the contact angle 114.6º can be
achieved only with a significant load on the bearing and small radial
clearances, as is known from the literature and from the works of the author
[20, 21, 23]. The contact angle = 180º is not
achieved even at zero clearance. In contrast, in MP plain bearings, the use of
these techniques is unreasonable given the significant difference between the
Young's modulus of the steel shaft and the composite bushing, as mentioned
above.
The contact pressures p and рmax in the considered MP bearings are estimated: = 5000 N: р0 = 4.94 – 6.34 (by Eq. (4)), р = 2.0 MPa
(by Eq. (13)), рmax = 2.55 MPa (by Eq. (14)); = 3000 N:
р0 = 3.5
– 4.89 (by Eq. (4)), р = 1.2 MPa
(by Eq. (13)), рmax = 1.53 MPa (by Eq. (14)). That is, according to
the conditional method of calculation of bearing capacity without taking into
account the type of polymeric material in the bearing, there will be much lower
contact pressures than determined by the above author's method of calculation.
Fig. 10 shows the Young's modulus of unfilled (base) polyamides and
polyamide-based composites established by the Oliver - Farr method.
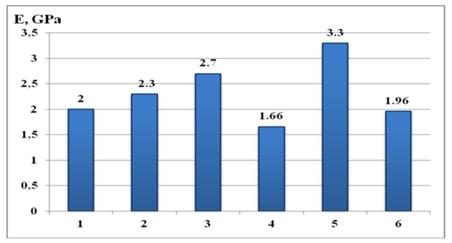
Fig. 10. Modulus of elasticity of
polyamides and polyamide composites
Different polyamide fillers PA6 have different
effects on the Young's modulus of composites. Fibreglass and carbon fibre
increase it, molybdenum disulfide reduces it, and oil has almost no effect on
it.
The methods of calculation of durability and
bearing capacity of MP bearings presented together with a technique of model
triboexperimental research of materials at sliding friction provide an
opportunity of their effective research at designing.
Program for calculations was developed in
PYTHON.
5.
CONCLUSIONS
The main research results are:
1.
For
polyamides widely used in MP bearings (PA6, PA66, PA6 + 30GF, PA6 + 30CF, PA6 +
MoS2, PA6 + oil) the wear resistance was experimentally investigated
according to the pin-on-disk friction scheme (ISO 7148-2) and wear resistance
indicators were determined. Wear resistance diagrams were plotted using them.
The wear resistance characteristics of polymeric materials as basic parameters
of their wear kinetics at dry sliding friction were determined. Due to the
modification of polyamide PA6 with different fillers, its wear resistance
increased almost threefold (oil filled cast polyamide PA6 + oil).
2.
The influence
of load, wear resistance and friction coefficient, Young's modulus on the
durability of the bearing and its contact parameters was investigated. It was
established that the durability of the MP bearing depends on the complex effect
of these three characteristics, and not only on the wear resistance of the
bushing polymeric material. It was found that the durability of the
bearing with a bushing made of filled composites increases and was three times
greater than for the composite PA6 + MoS2 than PA6.
3.
It was
established that at the same loads on the bearing, the contact parameters
differed markedly depending on the type of polyamide. This was due to their
different elastic properties (rigidity), which are characterized by the Young's
modulus.
4. Quantitative and qualitative regularities of the
influence of contact pressure on the coefficient of sliding friction in the
investigated metal – polymer tribocouples were established. The confirmation of
the general regularity of its significant decrease upon an increase in contact
pressure in an experimental tribocouple was obtained.
5. A typical method of calculating contact
pressures gives a significant understatement for MP bearings (in 2.5… 4.1
times) depending on the load.
6. One important property of the developed methods
for calculating the durability and bearing capacity of MP bearings was that the
solution to the problem is presented in a closed form, which allows its
practical implementation to be carried out using simple software tools,
starting from Microsoft Excel. Based on these methods, it was also possible to
carry out, when designing bearings, an optimization according to the criteria
of durability, contact strength, wear, as well as the optimal choice of
materials.
7.
The results of the research
can be used in practice to optimize the choice of polyamides in moving sliding
friction assemblies of vehicles in terms of load-bearing capacity, wear
resistance, and durability.
8.
In particular, polyamides of
the studied type were used in the engine connecting rod bearing [32], clutch
discs [38], shaft-bushing bearing of an automotive turbocharger wastegate
system [39], for the bearing of an automatic car belt tensioner [40].
References
1.
Chernets M.
2000. Contact-friction strength and
durability of tribotechnical sliding systems. Lublin University of
Technology, Lublin.
2.
Goryacheva
I.G. 2001. Mechanics of frictional
interaction. Nauka, Moscow.
3.
Kuzmenko
A.G. 2002. Methods for the Numerical
Analysis of Wear and Reliability. Podillya Technological University, Khmelnytsky.
4.
Kuzmenko
A.G. 2010. Development of methods of
contact tribomechanics. KhNU, Khmelnytsky.
5.
Dykha O., O. Babak, M. Dykha. 2017. The
wear models of cylindrical sliding tribosystems. Actual problems of modern
science. Bydgoszcz, Poland.
6.
Dykha A.,
D. Marchenko. 2018. Prediction the wear of sliding bearings. International Journal of Engineering &
Technology 7(2): 4-8. DOI: http://dx.doi.org/10.14419/ijet.v7i2.23.11872.
7.
Sorokatyi
R., M. Chernets, A. Dykha, O. Mikosyanchyk. 2019. Phenomenological model of
accumulation of fatigue tribological damage in the surface layer of materials. Mechanisms and Machine Science 73:
3761-3769. DOI: https://doi.org/10.1007/978-3-030-20131-9_371.
8.
Zwieżycki W. 1999. Prognozowanie niezawodności zużywających się elementów maszyn. [In Polish: Forecasting the reliability of wearing machine elements]. ITE,
Radom.
9.
Sorokatyi
R.V. 2002. Modelling the behaviour of tribosystems using the method of
triboelements. Journal of Friction and
Wear 23(1): 16-22.
10.
Sorokatyi
R.V. 2003. Solution of the problem of wear of a fine elastic layer with a rigid
bearing mounted on a rigid shaft using the method of triboelements. Journal of Friction and Wear 24(1):
35-41.
11.
Wielieba W. 2013. Bezobsługowe łożyska ślizgowe z polimerów termoplastycznych. [In Polish: Maintenance-free plain bearings made of thermoplastic polymers].
Politechnika Wrocławska, Wrocław.
12.
Rezaei A., W.
Ost, W.V. Paepegem, P. De Baets, J. Degrieck. 2011. Experimental study and numerical simulation of
the large-scale testing of polymeric composite journal bearings:
Three-dimensional and dynamic modelling. Wear
270: 431-438. DOI: https://doi.org/10.1007/s11249-009-9518-3.
13.
Rezaei A., W.
Ost, W. Van Paepegem, P. De Baets, J. Degrieck. 2012. A study on the effect of the clearance on the
contact stresses and kinematics of polymeric composite journal bearings under
reciprocating sliding conditions. Tribology
International 48: 8-14. DOI: https://doi.org/10.1016/j.triboint.2011.06.031.
14.
Rezaei A., W.
Ost, W. Van Paepegem, P. De Baets, J. Degrieck. 2012. Adaptive finite element simulation of wear
evolution in radial sliding bearing. Wear
296(1-2): 660-671. DOI: https://doi.org/10.1016/j.wear.2012.08.013.
15.
Bely V.A., A.I. Sviridenok, M.I.
Petrokovets, V.G. Savkin. 1982. Friction and wear in polymer – based
materials. Pergamon Press, Oxford.
16.
Myshkin
N.K., M.I. Petrokovets, A.V. Kovalev. 2005. Tribology of polymers: Adhesion,
friction, wear, and mass-transfer. Tribology
International 38: 910-921. DOI: https://doi.org/10.1016/j.triboint.2005.07.016.
17.
Myshkin N.,
A. Kovalev, D. Spaltman. 2014. Contact mechanics and tribology of polymer
composites. J Appl Polym Sci 131:
39870. DOI: https://doi.org/10.1002/app.39870.
18.
Myshkin N.,
A. Kovalev. 2018. Adhesion and surface forces in polymer tribology –
A review. Friction 6: 143-155.
DOI: https://doi.org/10.1007/s40544-018-0203-0.
Chernets M.V., I.N. Gonchakivskii, Yu. Skvarok. 1987. Solution of a Contact Problem for Cylindrical Bodies with Faceted Configuration and with Internal Contact. 19(6):-https://doi.org/10.1007/BF01522847.
20.
Chernets M.,
M. Pashechko, A. Nevchas. 2001. Methods of forecasting and increasing the wear
resistance of tribotechnical sliding systems. In 3 volumes. Vol. 1. Research and calculation of sliding
tribosystems, methods to increase durability and wear resistance. KOLO,
Drogobich.
21.
Chernets
M.V. 2013. Tribocontact problems for
cylindrical joints with technological roundness. Lublin University of
Technology, Lublin.
22. Chernets M.V., O.E. Andreikiv, N.M. Liebiedieva,
V.B. Zhydyk. 2009. A model for evaluation of wear and durability of plain
bearing with small out-of-roundness. Materials
Science 3: 279-290. DOI: https://doi.org/10.1007/s11003-009-9176-5.
23.
Chernets
M.V. 2009. Сontact
problem for a cylindrical joint with technological faceting of the contours of
its parts. Materials Science 6:
859-868. DOI: https://doi.org/10.1007/s11003-010-9252-x.
24.
Chernets
M., Ju. Chernets. 2015. Generalized method for calculating the durability of
sliding bearings with technological out-of-roundness of details. Proc. IMechE. Part J: Journal of Engineering
Tribology 229(2): 216-226. DOI: https://doi.org/10.1177/1350650114554242.
25.
Chernets
M.V. 2015. Prediction of the life of a sliding bearing based on a cumulative
wear model taking into account the lobing of shaft contour. Journal of Friction and Wear 36(2):
163-169. DOI: https://doi.org/10.3103/S1068366615020038.
26.
Chernets
M.V., S.V. Shil’ko, M.I. Pashechko, M. Barshch. 2018. Wear resistance of glass-
and carbon-filled polyamide composites for metal-polymer gears. Journal of Friction and Wear 39(5):
361-364. DOI: https://doi.org/10.3103/S1068366618050069.
27. Chernets M., J. Chernets, M. Kindrachuk, A.
Kornienko. 2020. Methodology
of calculation of metal-polymer sliding bearings for contact strength,
durability and wear. Tribology in
Industry 42(4): 572-584. DOI: https://doi.org/10.24874/ti.900.06.20.10.
28.
Palabiyik
M., S. Bahadur. 2002. Tribological studies of polyamide 6 and high-density рolyethylene blends filled with PTFE and copper
oxide and reinforced with short glass fibers. Wear 253: 369-376. DOI: https://doi.org/10.1016/S0043-1648(02)00144-8.
29.
Feyzullahoglu
E., Z. Saffak. 2008. The tribological behaviour of different engineering
plastics under dry friction conditions. Materials
and Design 29: 205-211. DOI: http://dx.doi.org/10.1016/j.matdes.2006.11.012.
30.
Ünlü B.S., E.
Atik, S. Köksal. 2009. Tribological
properties of polymer-based journal bearings. Mater. Design. 30(7): 2618-2622. DOI: https://doi.org/10.1016/j.matdes.2008.11.018.
31.
Turan
Demirci M., H. Düzcükoğlu. Wear behaviours of PTFE reinforced PA 66. Journal bearings. International scientific
conference. 19-20 November, 2010. Gabrovo, Bulgaria. P. 249-253.
32.
Mastan V., V.
Raja Kiran Kumar, Ch. Kiran Kumar. 2012. Study
of Friction and Wear on Journal Bearings. International
Refereed Journal of Engineering and Science 1(4): 63-70.
33. Kalácska G. 2013. An engineering approach to dry
friction behaviour of numerous engineering plastics with respect to the
mechanical properties. Express Polymer
Letters 7(2): 199-210. DOI: http://dx.doi.org/10.3144/expresspolymlett.2013.18.
34.
Mithun Kulkarni V., K. Elangovan, K.
Hemachandra Reddy, S.J. Basappa. 2016.
Tribological behaviours of ABS and PA6 polymer metal sliding combinations under
dry friction, water absorbed and electroplated conditions. Journal of Engineering Science and Technology 11(1): 068-084.
35.
Pogaˇcnik A., A. Kupec, M. Kalin. 2017. Tribological properties of polyamide (PA6)
in self-mated contacts and against steel as a stationary and moving body. Wear 378-379: 17-26. DOI: https://doi.org/10.1016/j.wear.2017.01.118.
36. Jozwik
J., K. Dziedzic, M. Barszcz, M. Pashechko. 2020. Analysis and Comparative Assessment of Basic
Tribological Properties of Selected Polymer Composites. Materials 13(1): 75. DOI: https://doi.org/10.3390/ma13010075.
37.
Budynas R.G., Nisbett J.K. 2019. Shigley’s
Mechanical Engineering Design.
McGraw-Hill, New York.
38.
De Almeida Rosa A.G., J.A.
Moreto, M.D. Manfrinato, L.S. Rossino. 2014. Study
on friction and wear behaviour of SAE 1045 steel, reinforced nylon 6.6 and NBR
rubber used in clutch disks. Mat. Res. 17(6): 1397-1403. DOI: https://doi.org/ 10.1590/1516-1439.282714.
39. Schmidt A.A., T. Schmidt, O. Grabherr, D. Bartel. 2018. Transient wear simulation based on three-dimensional finite element analysis for a dry running tilted shaft-bushing bearing. Wear 408-409: 171-179. DOI: https://doi.org/10.1016/j.wear.2018.05.008.
40. Fortes R.R., J.R. de Camargo, G.E.O.
Giacaglia, W. de Queiróz Lamas. 2021. Tribological study of a polymer bushing wear
with a steel axle for an automatic car tensioner. Proc. IMechE. Part J: Journal
of Engineering Tribology 236. DOI: https://doi.org/10.1177/13506501211053514.
Received 20.02.2024; accepted in revised
form 10.05.2024
Scientific
Journal of Silesian University of Technology. Series Transport is licensed
under a Creative Commons Attribution 4.0 International License