Article
citation information:
Akgüngör, A.P.,
Mercan, E.Z. An analysis of Type I
dilemma zone at signalised intersections. Scientific Journal of Silesian University of
Technology. Series Transport. 2021, 112,
05-16. ISSN: 0209-3324. DOI: https://doi.org/10.20858/sjsutst.2021.112.1.
Ali Payıdar AKGÜNGÖR[1], Elif Zahide MERCAN[2]
AN
ANALYSIS OF TYPE I DILEMMA ZONE AT SIGNALISED INTERSECTIONS
Summary. Intersections, for
vehicles coming from different directions, are conflict points in road
networks. When a driver approaching a signalised intersection encounters the
yellow light, he/she is in a dilemma either to safely stop or to pass through
the intersection during clearance time. The decision to stop or to pass may
change depending on some factors such as duration of yellow light, deceleration
and acceleration rate, width of intersection, speed and length of vehicle, etc.
This study aims to put forth the effects of some related factors affecting the
length of the Type I dilemma zone. To perform this study, five factors
including vehicle speed, maximum deceleration rate, perception-reaction time,
clearance time, the total intersection width-vehicle length were considered and
a total of 648 different traffic cases were investigated. The study results
showed that the Type I dilemma zone length increased with the increase of
speed, total intersection width-vehicle length and perception-reaction time,
but decreased with the increase of clearance time and deceleration rate.
Keywords: dilemma zone, signalised intersections, stop or
go decision
1. INTRODUCTION
Intersections are potential accident areas in
road networks because two or more traffic streams cross or merge on the
approach. To provide a safety manoeuvre
movement for both vehicles and pedestrian traffic at the intersection, they are
signalised with optimum signal time based on
time-sharing principles. Although traffic signalisation
mostly prevents traffic accidents at intersections, collisions occur for
various reasons such as driver behaviours, speed, the
duration of perception-reaction time and yellow time. As drivers approach a signalised intersection, when the traffic signal changes
from green to yellow, travelling vehicles stay in a zone where they have to
decide to stop or go at the intersection to prevent a potential right-angle
collision or rear-end collision. Gazis et al. first
introduced this safety problem at signalised
intersections to traffic engineering literature as a dilemma zone problem. They
defined the dilemma zone (DZ) as a zone that vehicles at the onset of the yellow
indication neither stop safely nor pass clearly through the intersection before
the stop line [9]. This concept as proposed by Gazis
et al. is also known as the Type I dilemma zone [17, 29, 30].
The reason for the Type I dilemma zone is associated with inappropriate signal
timing. Especially, insufficient yellow or clearance time (yellow time + all
red time) causes the Type I dilemma zone. On the other hand, it has been recognised that longer yellow or clearance intervals could
eliminate the Type I dilemma zone; however, it would lead to longer option
zones where drivers are indecisive to stop or cross at the onset of yellow
light. This option zone is called the Type II dilemma zone or “Indecision
zone” [15]. The Type II dilemma zone was formally proposed by the
Southern Section of ITE and included in the technical
committee report in 1974 [12]. Several studies have been conducted to define
the location of the Type II dilemma zone based on the possibility of stopping [3,
20, 31, 32]. Zegeer and Deen
suggested an approach to defining the boundaries of the Type II dilemma zone
depending on the driver’s decision-making. They expressed the dilemma
zone as a zone where more than 10% and less than 90% of drivers would choose to
stop at the start of the yellow signal [32].
The Type I and Type II dilemma zones
are depicted in Figure 1. Here, XC
is the minimum distance from the stop line where a vehicle can safely stop
before the stop line, and X0 is the maximum distance from the stop line
where a vehicle can cross and clear the intersection by the end of the
clearance time. In their study, Gazis et al. derived
the values of XC and X0 given
in Equations 1 and 2 [9].
![]() (1)
(1)
![]() (2)
(2)
where:
XC is minimum stopping distance (m), V0 is
vehicle speed onsetting yellow time (m/s), tr is
driver’s perception- reaction time (sec), dmax is maximum
deceleration rate of vehicle (m/s2), X0 is the maximum safety
crossing distance (m), τ is
yellow time (sec), W is the
intersection width (m), L is average
vehicle length (m), amax
is the maximum acceleration rate of vehicle (m/s2).
If a driver keeps his vehicle speed
without accelerating while approaching the intersection when he encounters the
yellow light, Equation 3 can be expressed as follows.
![]() (3)
(3)
![]() (4)
(4)
where:
tC is clearance time (sec) consisting
of the sum of yellow time and all red time, ty
is yellow time and tAll Red is safety interval for
all directions.
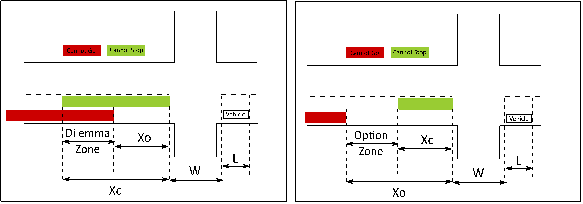
(a)
(b)
Fig. 1. a. The Type I dilemma
zone b. Type II dilemma
(option) zone
As seen from Figure 1, when XC is greater than X0,
the vehicle physically locates somewhere between XC and X0 and the Type 1 dilemma zone exists. On the
other hand, when X0
is greater than Xc, then as the clearance time passing distance is
greater than the minimum stopping distance, the vehicle is within an option
zone. It means the driver can either stop safely or proceed through the
intersection before the end of clearance time. Regardless of the decision, the
driver can make it.
Different factors, such as vehicle speed,
distance to intersection, the size of vehicle, driver’s
perception-reaction time, the length of yellow time, etc., affect the driver behaviour and the length of the dilemma zones. Besides, the
existence of the dilemma zone is also effective on the performance and safety
of the intersection. Therefore, the analysis of the factors that could affect
the dilemma zone has been one important issue of traffic engineering.
2. INFLUENCING FACTORS TO DILEMMA ZONE LENGTHS
Traffic is a dynamic process in nature, thus,
many factors affect drivers’ behaviour. Each
driver approaching a signalised intersection may react differently
on seeing the yellow signal. Given decisions may change based on driver behaviours, traffic flow conditions, intersection and
vehicle characteristics [2, 4, 5, 21]. Among them,
driver behaviour plays an important role in the
drivers’ decision-making process and intersection safety [11, 19, 24].
Some drivers are aggressive and prone to
accidents while others are calm, obeying the rules. Two parameters, such as
perception-reaction time and acceleration/deceleration rate, affect the driving
performance of most drivers. Many studies have investigated the effects of
these two parameters on traffic flow [6-8, 10, 16, 26, 28].
However, the parameters of the Type I dilemma zone like perception-reaction time and
acceleration/deceleration rate are assumed constant value. ITE
recommended 3.05 m/s2, 0 m/s2 and 1.0 sec, respectively, for deceleration,
acceleration and perception-reaction time values [13]. The design standards of
the American
Association of State Highway and Transportation Officials (AASHTO) also defined 3.41 m/s2,
0 m/s2 and 1.5 sec, respectively, as the
default value of deceleration, acceleration and perception time [1]. Although Gazis et al., considered acceleration rate as a linear
function of speed, acceleration rate in most studies generally was assumed
constant as 0 m/s2. Saito et al.
investigated the relation between deceleration rate and stopped vehicles. The
study result indicated that the range for deceleration rate was between 1.5 and
6 m/s2. The average value of 3.6 m/s2 was in 50 percentile value. In the same
study, the average reaction time for stopping ranged approximately 1-2 sec. Rakha et al. (2007) performed a field study to determine
the average perception-reaction time and expressed that observed samples ranged
from 0.3 to 1.7 sec.
Vehicle speed, distance from stop line, the
length of intersection, duration of yellow and clearance time are other
parameters that affect driver decisions in the dilemma zones. Hence, the length
and type of dilemma zones can be varied based on decisions by the drivers [25].
However, the studies by Urbanik and Koonce, and Si et al. showed that the Type I dilemma zone
is eliminated by adequate yellow and clearance times [27, 30]. Saito et al.
obtained similar results related to the Type I dilemma zone with their
observation study before Urbanik and Koone’s study [25]. According to the study results,
as yellow and clearance times increased, vehicle rate in the Type I dilemma
zone decreased but increased in the option zone. Rakha
et al. stated that when the yellow light is turned on, in the case that a
vehicle travelled at high speed and it was a close distance to the stop line,
the stopping probability of that vehicle at the intersection was low. They also
pointed out that most drivers would not stop at the intersection if the time to
the intersection is 1.6 s at the beginning of the yellow interval [23]. Puan and
Ismail posited that most drivers were reluctant to stop at the onset of the
yellow time. On the other hand, they expressed that most drivers tend to stop
at the beginning of the yellow interval, if travelling at low speed,
approaching a large and sophisticated intersection, having a long distance to
stop line, and driving in heavy traffic [22].
Besides the above factors, other potential
factors such as vehicle type, adverse weather, driver experience, gradient,
pavement conditions, position in a platoon, signal control type, pedestrian behaviours, existence of
countdowns can affect driver behaviour in a dilemma
zone [19, 33].
3.
ANALYTIC STUDY OF THE DILEMMA ZONE FOR SIGNALISED
INTERSECTIONS
The control of an intersection for safe
crossing can be provided by the signalisation system.
However, the deficiency of signalisation at
high-speed signalised intersections where the speed
limit is greater than 65 km/h dilemma zone may cause problems, which may result
in rear-end and right-angle crashes. As explained above, several factors affect
the length and type of dilemma zone. Subsequently, an analytical study was
conducted employing Equations 1 and 3 to find out the effectiveness of the
influencing factors on the dilemma zone. In the study, vehicle speeds V0 were changed from 50 km/h (13.89 m/s)
to 100 km/h (27.78 m/s) with 10 km/h (2.78 m/s) increments while the
clearance times of the intersection were varied from 3 to 6 seconds. Selected
clearance times for this study are plausible, as the Manual on Uniform Traffic Control
Devices recommends that the minimum and maximum yellow time are 3 and 6
seconds, respectively [18]. In addition, the Manual of Traffic Signal Design emphasises that a clearance time greater than 6 seconds be
carefully examined before being implemented [14].
Human perception-reaction time
varies between 0.8 and 2.5 seconds depending on age, sex, visibility,
concentration and the environmental conditions at the time of response. While
this period increases with alcohol use and fatigue, it can decrease with
experience and focused attention. For signal timing purposes, the ITE recommends a perception-reaction time of 1.0 second for
simplicity [13]. AASHTO allows 1.5 seconds for
perception time and 1.0 second for reaction time [1]. Some studies suggest that
the upper range of perception-reaction time begins at 0.78 second in the
laboratory environment and become 2.50 seconds in an urban street in real-time.
Therefore, the driver’s perception-reaction time tr ranges from 1 to 2 seconds with
0.5 sec. increments in this study.
The maximum deceleration rate of vehicle d max
is an important factor in determining the boundaries of dilemma zones, and
varies from vehicle to vehicle, depending on vehicle speeds and
characteristics. The value of maximum deceleration rate is assumed to be
constant as 3.41 m/s2 (11.2
ft/s2) by AASHTO and 3.05 m/s2
(10 ft/s2)
by the ITE (1, 9). Therefore, maximum deceleration
rate of vehicle is assumed 2, 3, 4 m/s2 in
this work.
When a driver approaching a signalised
intersection encounters the yellow light within the dilemma zone, his or her
decision of passing or not passing the intersection may vary depending on his
or her vehicle speed, the size of the intersection, distance to the stop line
and the length of the vehicle he or she uses. Drivers usually tend to stop at
the beginning of the yellow light at complex intersections consisting of 3 or
more lanes while they tend to proceed during clearance interval at the smaller
intersections. In analysing the dilemma zone, both
small and large intersection considered the effect of intersections, the sum of
intersection width W and the average vehicle length L as
15, 25 and 35 m, respectively.
In the analysis of the dilemma zone, a total of
6x4x3x3x3= 648 different traffic cases were
considered and their interaction among them was investigated. The parameters
and their values examined are given in Table 1.
Tab. 1
Effective parameters used in the dilemma zone
calculations and their values
|
Speed (V0) (km/h)/(m/s) |
Clearance time tc (s) |
Maximum deceleration rate dmax (m/s2) |
Perception-reaction time tr (s) |
Total length of intersection and
vehicle W (m) |
|
50/13.89 |
3 |
2 |
1 |
15 |
|
60/16.67 |
4 |
3 |
1.5 |
25 |
|
70/19.45 |
5 |
4 |
2 |
35 |
|
80/22.22 |
6 |
|
|
|
|
90/25.00 |
|
|
|
|
|
100/27.78 |
|
|
|
|
Dilemma and option zones for 648 various
traffic cases were calculated using Equations 1 and 3. Tables 2 and 3 show the
relationship among various speed, clearance time and the intersection width
plus vehicle length on dilemma zones for the same deceleration rate and
perception-reaction time (a=3 m/s2 and tr=1.5 s).
Tab. 2
The relation between speed and
clearance time with W=15 m for the
dilemma zone
(a=3
m/s2 and tr=1.5 s)
|
|
tc =3 s |
tc =4 s |
tc =5 s |
tc =6 s |
||||||||
|
Speed (km/h) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
|
50 |
52.99 |
26.67 |
26.32 |
52.99 |
40.56 |
12.43 |
52.99 |
54.45 |
-1.46 |
52.99 |
68.34 |
-15.35 |
|
60 |
71.31 |
35.00 |
36.30 |
71.31 |
51.67 |
19.63 |
71.31 |
68.34 |
2.97 |
71.31 |
85.01 |
-13.70 |
|
70 |
92.19 |
43.34 |
48.86 |
92.19 |
62.78 |
29.41 |
92.19 |
82.23 |
9.96 |
92.19 |
101.68 |
-9.48 |
|
80 |
115.65 |
51.67 |
63.98 |
115.65 |
73.90 |
41.76 |
115.65 |
96.12 |
19.53 |
115.65 |
118.34 |
-2.69 |
|
90 |
141.69 |
60.01 |
81.68 |
141.69 |
85.01 |
56.68 |
141.69 |
110.01 |
31.68 |
141.69 |
135.01 |
6.67 |
|
100 |
170.29 |
68.34 |
101.95 |
170.29 |
96.12 |
74.17 |
170.29 |
123.90 |
46.39 |
170.29 |
151.68 |
18.61 |
Tab. 3
The relation between speed and
clearance time with W=35 m for the
dilemma zone
(a=3
m/s2 and tr=1.5 s)
|
|
tc =3 s |
tc =4 s |
tc =5 s |
tc =6 s |
||||||||
|
Speed (km/h) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
Xs (m) |
X0 (m) |
DZ (m) |
|
50 |
52.99 |
6.67 |
46.32 |
52.99 |
20.56 |
32.43 |
52.99 |
34.45 |
18.54 |
52.99 |
48.34 |
4.65 |
|
60 |
71.31 |
15.00 |
56.30 |
71.31 |
31.67 |
39.63 |
71.31 |
48.34 |
22.97 |
71.31 |
65.01 |
6.30 |
|
70 |
92.19 |
23.34 |
68.86 |
92.19 |
42.78 |
49.41 |
92.19 |
62.23 |
29.96 |
92.19 |
81.68 |
10.52 |
|
80 |
115.65 |
31.67 |
83.98 |
115.65 |
53.90 |
61.76 |
115.65 |
76.12 |
39.53 |
115.65 |
98.34 |
17.31 |
|
90 |
141.69 |
40.01 |
101.68 |
141.69 |
65.01 |
76.68 |
141.69 |
90.01 |
51.68 |
141.69 |
115.01 |
26.67 |
|
100 |
170.29 |
48.34 |
121.95 |
170.29 |
76.12 |
94.17 |
170.29 |
103.90 |
66.39 |
170.29 |
131.68 |
38.61 |
As seen from the Tables above, it is realised that the Type I dilemma zone length increases with
the increase of speed but decreases with the increase of clearance time.
Additionally, an increase in clearance time causes the Type 1 dilemma zone to
disappear while the dilemma zone of Type 2, known as the stop or go decision
zone, arises (Table 2). At the same time, an increase in the width of the
intersection also causes an increment in the length of the Type I dilemma zone
(Figure 3).
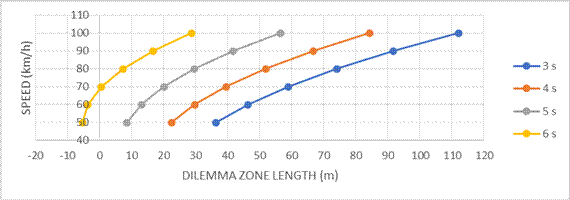
Fig. 2. The relation between speed
and clearance time with W=25 m for
the dilemma zone
(a=3
m/s2 and tr=1.5 s)

Fig. 3. The relation between speed
and intersection width and dilemma zone length
(tc
=4, a=3 m/s2
and tr=1.5
s)
Tab. 4
The relation between speed and
deceleration rate with tr =
1 sec for the dilemma zone
(tc
=3 s, W=25 m)
|
|
a =2 m/s2 |
|
a =3 m/s2 |
|
a =4 m/s2 |
||||||
|
Speed (km/h) |
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
50 |
62.12 |
16.67 |
45.45 |
|
46.05 |
16.67 |
29.38 |
|
38.01 |
16.67 |
21.34 |
|
60 |
86.12 |
25.00 |
61.12 |
|
62.97 |
25.00 |
37.97 |
|
51.40 |
25.00 |
26.39 |
|
70 |
113.98 |
33.34 |
80.64 |
|
82.47 |
33.34 |
49.13 |
|
66.71 |
33.34 |
33.38 |
|
80 |
145.70 |
41.67 |
104.03 |
|
104.54 |
41.67 |
62.87 |
|
83.96 |
41.67 |
42.29 |
|
90 |
181.28 |
50.01 |
131.27 |
|
129.19 |
50.01 |
79.18 |
|
103.14 |
50.01 |
53.13 |
|
100 |
220.71 |
58.34 |
162.37 |
|
156.40 |
58.34 |
98.06 |
|
124.25 |
58.34 |
65.91 |
Tab 5.
The relation between speed and
deceleration rate with tr =
2 s for the dilemma zone
(tc
=3 s, W=25 m)
|
|
a =2 m/s2 |
|
a =3 m/s2 |
|
a =4 m/s2 |
||||||
|
Speed (km/h) |
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
50 |
76.01 |
16.67 |
59.34 |
|
59.94 |
16.67 |
43.27 |
|
51.90 |
16.67 |
35.23 |
|
60 |
102.79 |
25.00 |
77.79 |
|
79.64 |
25.00 |
54.64 |
|
68.06 |
25.00 |
43.06 |
|
70 |
133.43 |
33.34 |
100.09 |
|
101.92 |
33.34 |
68.58 |
|
86.16 |
33.34 |
52.82 |
|
80 |
167.92 |
41.67 |
126.25 |
|
126.77 |
41.67 |
85.09 |
|
106.19 |
41.67 |
64.51 |
|
90 |
206.28 |
50.01 |
156.27 |
|
154.19 |
50.01 |
104.18 |
|
128.14 |
50.01 |
78.14 |
|
100 |
248.49 |
58.34 |
190.15 |
|
184.18 |
58.34 |
125.84 |
|
152.03 |
58.34 |
93.69 |
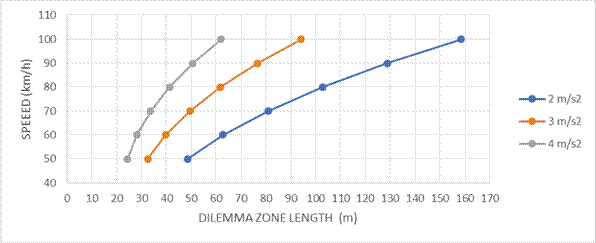
Fig. 4. The relation between speed,
deceleration rate and the dilemma zone
(tc
=4 s, tr
= 2 s, W=35 m)
It is noted that the Type I dilemma zone length
enlarges with the increase of speed yet decreases with the increase of
deceleration rate, as shown in Figure 4, Tables 4 and 5. Moreover, an increase
in perception-reaction time leads to an increase in the length of the Type 1
dilemma zone under the same intersection width and clearance time.
Tab. 6
The relation between clearance time
and perception-reaction time with V0 = 60 km/h for the dilemma zone (a =3 m/s2,
W=35 m)
|
|
tr = 1 s |
|
tr = 1.5 s |
|
tr = 2 s |
||||||
|
Clearance Time (s) |
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
3 |
62.97 |
15.00 |
47.97 |
|
71.31 |
15.00 |
56.30 |
|
79.64 |
15.00 |
64.64 |
|
4 |
62.97 |
31.67 |
31.30 |
|
71.31 |
31.67 |
39.63 |
|
79.64 |
31.67 |
47.97 |
|
5 |
62.97 |
48.34 |
14.63 |
|
71.31 |
48.34 |
22.97 |
|
79.64 |
48.34 |
31.30 |
|
6 |
62.97 |
65.01 |
-2.04 |
|
71.31 |
65.01 |
6.30 |
|
79.64 |
65.01 |
14.63 |
Tab. 7
The relation between clearance time
and perception-reaction time with V0 = 90 km/h for the dilemma zone (a =3 m/s2,
W=35 m)
|
|
tr = 1 s |
|
tr = 1.5 s |
|
tr = 2 s |
||||||
|
Clearance Time (s) |
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
Xs (m) |
X0 (m) |
DZ (m) |
|
3 |
129.19 |
40.01 |
89.18 |
|
141.69 |
40.01 |
101.68 |
|
154.19 |
40.01 |
114.18 |
|
4 |
129.19 |
65.01 |
64.18 |
|
141.69 |
65.01 |
76.68 |
|
154.19 |
65.01 |
89.18 |
|
5 |
129.19 |
90.01 |
39.18 |
|
141.69 |
90.01 |
51.68 |
|
154.19 |
90.01 |
64.18 |
|
6 |
129.19 |
115.01 |
14.17 |
|
141.69 |
115.01 |
26.67 |
|
154.19 |
115.01 |
39.18 |
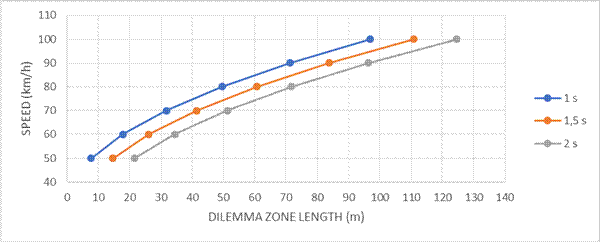
Fig. 5. The relation between
speed, perception-reaction time and the dilemma zone
(tc = 5 s, a =2 m/s2, W=15 m)
Tables 6 and 7 illustrate the relationship
between clearance time and perception-reaction time in the determination of the
dilemma zone. As seen from the Tables, the Type I dilemma zone length decreases
with an increase of clearance time. On the other hand, it also increases with
the increase of the perception-reaction time. This is not surprising, since
taken distance on the road is dependent not only on perception-reaction time
but also on the speed of the vehicle. This is the reason the dilemma zone
values in Table 7 are greater than those in Table 6. In addition, Figure 5
graphically depicts the impact of perception-reaction time. A look at Table 6
shows that due to enlarging clearance time, the Type I dilemma zone turns into
a decision zone (Type II dilemma zone). As mentioned before, while a longer
clearance time eliminates the Type I dilemma zone, it causes a longer decision
zone. This circumstance is coherent with other studies in the literature [25, 30].
4. CONCLUSIONS
When at a yellow light in a signalised
intersection, a driver faces the uncertainty of whether to stop or go through
the intersection. He or she decides this uncertainty. If his decision is
incorrect, it results in a red-light violation or a collision at the
intersection. Therefore, to reduce the dilemma zone problem and improve
intersection safety, many efforts have been proceeded by not only traffic
researchers but also decision-makers.
This study helps to better understand the
dilemma zone issue, especially the Type I dilemma zone concept. At the same
time, it investigates the relations among the factors affecting the dilemma
zone length. To analyse the relationships, 648
different traffic cases were considered. The study results showed that
increasing yellow time or clearance time eliminates the Type I dilemma zone but
increases the uncertainty in the driver’s decision, namely creating the
Type II dilemma zone. Further, the study indicated that the Type I dilemma zone
length increased with the increase of speed, perception-reaction time, the
intersection width (plus the length of vehicle) but decreased with an
increasing deceleration rate. For future dilemma zone studies, other factors,
such as traffic signal countdown timers, the effect of adverse weather
condition, gradient, advanced dilemma zone warning system, vehicle type,
vehicle position in traffic flow, should also be investigated.
References
1.
AASHTO,
2011. A Policy on Geometric Design of Highways and Streets, 6 th edition. American
Association of State Highway and Transportation Officials, Washington, D.C.
ISBN: 978-1-56051-508-1.
2.
Audu Akeem A.,
Olufemi F. Iyiola, Ayobami A. Popoola, Bamiji M. Adeleye, Samuel Medayese, Choene
Mosima, Nunyi Blamah. 2021. „The application of geographic information
system as an intelligent system towards emergency responses in road traffic
accident in Ibadan”. Journal of
Transport and Supply Chain Management 15(a546):
1-17. ISSN: 2310-8789.
3.
Bonneson James,Dan Middleton, Karl Zimmerman,
Hassan Charara, Montasir
Abbas, 2002. ,, Intelligent
detection-control system for rural signalized intersections.
Report No. FHWA /TX-02 /4022-2. Texas Department of Transportation,
Austin.Texas.
4.
Czech
Piotr. 2017. „Underage pedestrian
road users in terms of road accidents”.
In: Advances in Intelligent
Systems and Computing 505: 33-44. DOI: 10.1007/978-3-319-43991-4_4. Edited by: Sierpinski G. Intelligent transport systems
and travel behaviour. 13th Scientific
and Technical Conference on Transport Systems. Theory
and Practice (TSTP).
Katowice, SEP 19-21, 2016.
5.
Danilevičius Algimantas,
Marijonas Bogdevičius,
Modesta Gusarovienė, Gediminas Vaičiūnas, Robertas Pečeliūnas,
Irena Danilevičienė. 2018.
“Determination of Optimal Traffic Light Period Using a Discrete Traffic
Flow Model”. Mechanika 24(6): 845-851.
6.
Drozdziel
Pawel, Slawomir Tarkowski, Iwona Rybicka, Rafal Wrona, 2020. “Drivers’
reaction time research in the conditions in the real traffic” Open
Engineering 10(1): 35-47. DOI: https://doi.org/10.1515/eng-2020-0004.
7.
El-Shawarby Ihab, Hesham Rakha,
Vaughan Inman, Gregory Davis, 2007. “Evaluation of driver deceleration
behavior at signalized intersections”. Transportation Research Board
86th Annual Meeting. January 22-25, 2007. Washington D.C., U.S.A.
8.
El-Shawarby Ihab, Ahmed Amer, Hesham Rakha, 2008. “Evaluation
of driver stopping behavior on high-speed signalized intersection
approaches”, Transportation Research Board 87th Annual Meeting.
January 13-17, 2008. Washington D.C., U.S.A.
9.
Gazis Denos, Robert
Herman, Alexei Maradudin. 1960. “The problem of the
amber signal light in traffic flow”. Operations Research 8(1): 112-132.
DOI: https://doi.org/10.1287/opre.8.1.112.
10. Green
Marc. 2008. “How long does it take to stop? Methodological analysis of
driver perception-brake times”. Transportation Human Factors 2(3):
195-216. DOI: https://doi.org/10.1207/STHF0203_1.
11. Hichim Majid Farag,
Ahmed Shany Khusheef,
Saddam Hasan Raheemah. 2020.
“The Effects of Driver Age and Gender on Vehicle Stopping Distance Under Different Speeds”. European Transport \ Trasporti Europei 80(1): 1-11. ISSN 1825-3997. DOI: https://doi.org/10.48295/ET.2020.80.1.
12. ITE Technical Committee 18 (Chair: Parsonson Peter), 1974. “Small area detection at
intersection approaches”. Traffic Engineering 44(2): 8-17.
13. ITE.1999. Traffic
Engineering Handbook.Prentice Hall Englewood Cliffs.
ISBN: 0-935403-32-9.
14. Kell James H., Iris J. Fullerton. 1998. Manual of Traffic Signal Design. 3rd ed. Englewood Cliffs, NJ: Institute of Transportation Enginerering, Prentice-Hall. ISBN: 978-0135543382.
15. Machiani Sahar Ghanipoor,
Montasir Abbas. 2016. “Safety surrogate
histogram (SSH): A novel real-time safety
assessment of dilemma zone related conflicts at signalized intersections”.
Journal of Accident Analysis and Prevention 96: 361-370. DOI: https://doi.org/10.1016/j.aap.2015.04.024.
16. Magister
Tone, Rok Krulec, Milan
Batista, Leon Bogdanović. 2005. “The
driver reaction time measurement experiences”. In: Proceedings of the
7th Conference and Exhibition Innovative Automotive Technology IAT’05: 751-761. 21-22 April 2005. Bled,
Slovenia.
17. Moon J. Young, Fred Coleman. 2003.
“Dynamic dilemma zone based on driver behavior and car-following model at
highway – rail intersections”. Transportation Research Part B:
Methodological 37(4): 323-344. DOI:
https://doi.org/10.1016/S0191-2615(02)00014-0
18. MUTCD. 2009. The Manual on Uniform Traffic Control Devices
(MUTCD) for Streets and Highways,
2009 Edition. US Department
of Transportation the Federal Highway
Administrator.
19. Papaioannou Panagiotis. 2007.
“Driver behaviour dilemma zone and safety effects at urban signalised
intersections in Greece”. Accident Analysis & Prevention 39(1):
147-158. DOI: https://doi.org/10.1016/j.aap.2006.06.014.
20. Pawar Digvijay,
Dibyendu Pathak, Gopal Patil. 2020. „Modeling dynamic
distribution of dilemma zone at signalized
intersections for developing world
traffic”. Journal
of Transportation Safety
& Security. DOI: 10.1080/19439962.2020.1852464.
21. Prentkovskis Olegas,
Edgar Sokolovskij, Vilius Bartulis. 2010. “Investigating traffic accidents: a
collision of two motor vehicles”. Transport
25(2): 105-115.
22. Puan Othman Che, Che Ros Ismail.
2010. “Dilemma zone conflicts at isolated intersections controlled with
fixed-time and vehicle actuated traffic signal systems”. International Journal of Civil and Environmental Engineering 10(3): 19-25.
23. Rakha Hesham,Ihab El-Shawarby,Jose
Reynaldo, Setti. 2007. “Characterizing driver
behavior on signalized intersection approaches at the onset of a yellow-phase
trigger”. IEEE Transactions on Intelligent Transportation Systems 8(4):
630-640 DOI: 10.1109/TITS.2007.908146.
24. Rakha Hesham,Ahmed Amer,Ihab El-Shawarby. 2008. “Modeling driver behavior within a
signalized intersection approach decision-dilemma zone”.
Transportation
Research Record: Journal of the Transportation Research Board 2069(1): 16-25. DOI: https://doi.org/10.3141/2069-03.
25. Saito
TN. Ooyarna, Kojiro Sigeta. 1990. “Dilemma and option zones, the problem
and countermeasures-characteristics of zones, and a new strategy of signal
control for minimizing zones”. In: Proceedings of the Third
International Conference on Road Traffic Control. London, UK. 1-3 May 1990.
P. 137-141.
26. Setti Jose Reynaldo, Hesham
Rakha, Ihab El-Shawarby. 2007. “Analysis of brake
perception-reaction times on high-speed signalized intersection
approaches”. Transportation Research Board 86th Annual Meeting.
January 22-25, 2007.
Washington D.C., U.S.A.
27. Si
Jianwen, Thomas Urbanik,
Lee Han L. 2007. “Effectiveness of alternative detector configurations
for option zone protection on high-speed approaches to traffic signal”. Transportation
Research Record 2035(1): 107-113.
DOI: https://doi.org/10.3141/2035-12.
28. Taoka George. 1989. “Break reaction times
of unaltered drivers”. ITE Journal
59(3):
19-21.
29. Tarko Andrew, Wei Li, Luis Laracuente. 2006. “Probabilistic approach to
controlling dilemma occurrence at signalized intersections”. Transportation
Research Record: Journal of the Transportation Research Board 1973(1):
55-63.
DOI: https://doi.org/10.1177/0361198106197300107.
30. Urbanik Tom, Peter Coonce.
2007. “The dilemma with dilemma zones”. ITE
District 6th Annual Meeting. Portland, Oregon.
31. Zegeer
Charles. 1977.
“Effectiveness
of green-extension systems at high speed intersections”
Research Report 472. Kentucky Department of
Transportation, Lexington, Kentucky, USA.
32. Zegeer
Charles,Robert
Deen. 1978. “Green-extension systems at highspeed intersection”. ITE
Journal 48(11): 19-24.
33. Zhang
Yaping, Chuanyun Fu, Liwei Hu. 2014. “Yellow light dilemma zone researches
a review”. Journal of Traffic and Transportation Engineering 1(5):
338-352.
DOI: https://doi.org/10.1016/S2095-7564(15)30280-4.
Received 02.04.2021; accepted in revised form 30.05.2021
![]()
Scientific
Journal of Silesian University of Technology. Series Transport is licensed
under a Creative Commons Attribution 4.0 International License