Article
citation information:
Vakulenko, I.A., Plitchenko, S.,
Kurt, B., Proydak, S., Askerov, H. Transformation of structure during friction stir
welding. Scientific Journal of Silesian
University of Technology. Series Transport. 2021, 111, 181-191. ISSN: 0209-3324. DOI: https://doi.org/10.20858/sjsutst.2021.111.16.
Igor Alex VAKULENKO[1], Sergey
PLITCHENKO[2], Bulent
KURT[3], Svetlana PROYDAK[4],
Hangardas ASKEROV[5]
TRANSFORMATION
OF STRUCTURE DURING FRICTION
STIR WELDING
Summary. In comparison with low carbon steels, there is increased
interest in the use of aluminium-based alloys as materials for the manufacture
of welded structures rolling stock of railway transport. During friction stir
welding aluminium-based alloy, against the background of the analysis
structural transformations, issues of development hardening processes are
considered. Under conditions of existence, a temperature gradient at zone of
weld formation, shown degree approximation alloy to the conditions of
superplastic flow and influence from presence particles of the second phase on
grain size of matrix is estimated. Evaluation of the separate influence grain
size of matrix and state of solid solution at total hardness of the weld showed
dependence of their contributions on temperature of hot plastic deformation. As
the temperature of plastic deformation of alloy at area of the weld
increases, contribution to the total hardness from grain size increase and on
state of the solid solution decreases.
Keywords: chemical compound, grain size, hardness, recrystallisation, temperature
1. INTRODUCTION
The high activity of aluminium-based
alloys to oxygen atoms significantly limits their use for the manufacture of
structures using the electric arc welding technology. In the last few decades,
for the manufacture of welded structures from aluminium alloys in aviation [1],
automobile [2] mechanical engineering and railway transport [3], friction stir
welding (FSW) [4-7] has become widespread. A distinctive feature of this
technology is the absence of change at state aggregation of the metallic
material. The technology of FSW is based on the achievement of the area of the connect edges
high degree mixing of the metal material from the simultaneous action of hot
plastic deformation and rate of diffusion [8-10]. Indeed, if degree at mixing
of the metal and density of the weld is determined by the plastic properties,
the characteristics of strength and crack resistance would depend on the
development of structural transformation after finish active phase connected of
edges. For most aluminium alloys with a multiphase structure, the volume fraction,
dispersion and morphology of the phase components influence the development of
recrystallisation processes and the final grain size of the matrix. On the
other hand, most chemical compounds of aluminium alloys are partially
interacting with matrix [11-13], which involves their dissolution with
increasing heating temperature of the alloy. Consequently, with increasing
heating temperature and duration work tool, the greatest possible degree
plasticisation of the alloy for the implementation of FSW technology [14-16],
dissolution of the chemical compound should increase mobility boundaries of the
matrix grains with large angles of disorientation and increase size of grain [12,
14]. The above provisions are confirmed by the high sensitivity of stir friction
welding to the temperature and speed of hot plastic deformation [18]. Moreover,
small deviations from their optimal ratio significantly complicate the process
of forming a quality welded joint [9, 14, 19].
2. STATE OF PROBLEM
The special shape of the working tool ensures
transition of the metal along the entire thickness of the connecting edges in
such a plastic state, which should be sufficient for its quality mixing during
weld formation. With increasing temperature, decrease resistance to the propagation
of plastic deformation is not always accompanied by an increase at plasticity
of the metal material. This position is due to the implementation of a certain
ratio between the development of the hardening and softening processes during
hot deformation. To achieve high ductility, in addition to proposals to
maintain a uniform distribution of deformation [18, 19], it would, analysing
the mechanism of structural changes, achieve and maintain stable conditions for
the propagation of super plastic deformation at alloy. Further, in addition to
increasing the plastic properties by tens to hundreds times [19], there is a
decrease at deformation stress to abnormally low levels. In general, the
conditions for achieving a superplastic state correspond to the relationship:
![]() ,
(1)
,
(1)
where
s - deforming stress, m - a coefficient sensitivity to rate of
deformation, s1 - characteristic, which by absolute value and physical meaning
corresponds to stress of non-inverse motion of dislocations [20]. On the other
hand, the value s
has a certain dependence on the degree of plastic deformation (e):
![]() , (2)
, (2)
where:
K - coefficient of
proportionality, n - the exponent (coefficient of strain hardening [21]).
After
substitution (2) in (1), by the ratio:
![]() (3)
(3)
it
is possible to determine the contribution of strain hardening on deviation from
the achievement conditions of the superplastic state in metallic material.
Thus, when approaching the superplastic flow, the structural transformations
during hot deformation should compensate for the negative contribution of
deformation hardening. As you approach the specified state of the metal
material s
will be decrease, the corresponding decrease will be for s1 and n. Thus, when s1®0 and n@0, K will be equal to the operating stress. By the
relation (3) maximum value of m cannot exceed 0.5. In fact, given the high
sensitivity to small deviations from the optimal ratio parameters of hot
deformation (temperature, strain rate, grain size, invariance, etc.), this
characteristic will be at range 0<m<0,5. In addition to the temperature and deformation
conditions of the superplastic flow, the issues of achieving optimal structural
state metallic material and maintaining it constant during deformation period
are of great importance. At process of hot plastic deformation, effective
stress depends on the grain size (d) according to the ratio:
![]() ,
(4)
,
(4)
where:
a is an exponent (for most
metallic materials, it is approximately equal to 1 [22]), A is constant [23]. By (4) the decrease in s will be approach to superplastic state will be provided by a decrease
d. Then, it is quite reasonable to obtain a stable structure during hot
deformation, with the small grain of polyhedral shape [18, 21], in sizes to
several microns. The need to maintain a constant grain structure is due to a
change in the nature of the deformation distribution from grain size during the
superplastic flow. The smallest grains are predominantly deformed along near
boundaries with large angles of disorientation, at low level s. In large grains, the deformation is distributed throughout the
volume, which requires a continuous increase applied stress [20]. Such
different conditions can only be met by maintaining a certain ratio between the
hardening and the softening processes during hot plastic deformation. Indeed,
at temperatures below optimum value, when the plastic properties of the metal
become insufficient to achieve desired level of mixing, traces of the working
tool become coarser, with obvious signs of exfoliation dispersed fragments [15,
17]. Under conditions when the metal is overheated to
temperatures higher than optimal value, as shown in [14, 16], the zone
influence of thermal and region of the weld have strength properties of a
much lower level compared to the initial state. Then, preservation of the
partial influence from development of deformation hardening processes during
the propagation of plastic flow can be a factor of increasing strength of metal
in the weld to level by initial state [10, 14]. The question of mechanism
propagation of plastic deformation under conditions superplastic state remains
open. According to [9, 19], it is believed that over plastic flow should be
accompanied by rotation of randomly oriented grains relative direction of the
applied stress or alternation of slippage in volumes near the grain boundaries,
with subsequent migration of boundaries.
3. MATERIAL AND RESEARCH METHODS
As a material for
research, used alloy based on aluminium with a content of Mg 6.5%, Mn 0.85%, Fe
0.13%, a rest of Al. Plates 2.5 mm thick was butt welded by FSW technology, on
a specially designed research stand, using a working tool of known size and shape
of the shoulder and pin [8, 14, 19]. Investigations of the welding process were
carried out at different ratios of rotational speed working tool (![]() ) and force of its pressing to the connecting
edges (P). The speed of movement
working tool along connecting edges was constant and was 40 mm/min, the change
interval
) and force of its pressing to the connecting
edges (P). The speed of movement
working tool along connecting edges was constant and was 40 mm/min, the change
interval ![]() was
800-1600 min-1, and P was
100 - 1420 N. The temperature distribution (T)
by heating edges of the metal was measured using thermocouples such as chromel
- alumel, with location at different distances from the connecting edges. The
structure of the alloy was examined using a light microscope
"Epiquant". Size of grain alloy was determined by the use of methods
quantitative metallographic. Measurements of microhardness (Hm) were performed on a device
type PMT-3, under load on the indenter 0.05N. Measurement data Hm was used as a characteristic
of strength properties for micro volumes of the alloy.
was
800-1600 min-1, and P was
100 - 1420 N. The temperature distribution (T)
by heating edges of the metal was measured using thermocouples such as chromel
- alumel, with location at different distances from the connecting edges. The
structure of the alloy was examined using a light microscope
"Epiquant". Size of grain alloy was determined by the use of methods
quantitative metallographic. Measurements of microhardness (Hm) were performed on a device
type PMT-3, under load on the indenter 0.05N. Measurement data Hm was used as a characteristic
of strength properties for micro volumes of the alloy.
4. RESULTS AND DISCUSSION
In general, the process of FSW can
be divided into two successive stages. From the beginning, a pin and shoulder
are immersed at alloy under load, without moving the tool along the edges.
According to the scheme (Figure 1a), the action of pressing force for a certain
duration leads to a corresponding increase at heating temperature of the edges
on the opposite side from the surface shoulder of the instrument (Figure 1b). The first stage ends with
heating the edges to a temperature that provides the required level of plastic
properties for high-quality mixing of the metal. The second stage starts from
the moment the tool is moved along the connecting edges. In proportion to the
speed of the weld formation, the energy consumption for continuous heating of
cold volumes of metal first with the shoulder, and then with the tool pin leads
to the emergence gradient of temperature and decrease of average temperature on
the thickness of the edges. This is confirmed by the decrease in temperature
from extremes in Figure 1b. To analyse the combined effect of the main
technological parameters (P and ![]() ) on
heating temperature metal of the connecting edges, dependences P ~ f (T) were constructed using
experimental data
) on
heating temperature metal of the connecting edges, dependences P ~ f (T) were constructed using
experimental data ![]() (Figure 1a, b). According to the curves
(Figure 1c), it is determined that regardless of the value
(Figure 1a, b). According to the curves
(Figure 1c), it is determined that regardless of the value ![]() ,
moment of violation directly proportional relation P ~ f (T) corresponds to approximately the equal temperature of
90-95°C. Generally, it should be assumed
that at this temperature, the process of softening begins to compensate for a
significant part resistance of the edges to immersion of the tool
(strengthening alloy from hot plastic deformation). Indeed, with the increase
of hot deformation temperature, processes moving of dislocations on different
crystallographic sliding systems are significantly accelerated, which promotes
their annihilation and redistribution at alloy matrix [20]. As a result,
dislocation subgrains of polygonal shape are formed, separated by subboundaries
of different types, with different angles of disorientation [19, 22]. It is
experimentally determined that the sharp decrease at deforming force (Figure
1b), especially at elevated
,
moment of violation directly proportional relation P ~ f (T) corresponds to approximately the equal temperature of
90-95°C. Generally, it should be assumed
that at this temperature, the process of softening begins to compensate for a
significant part resistance of the edges to immersion of the tool
(strengthening alloy from hot plastic deformation). Indeed, with the increase
of hot deformation temperature, processes moving of dislocations on different
crystallographic sliding systems are significantly accelerated, which promotes
their annihilation and redistribution at alloy matrix [20]. As a result,
dislocation subgrains of polygonal shape are formed, separated by subboundaries
of different types, with different angles of disorientation [19, 22]. It is
experimentally determined that the sharp decrease at deforming force (Figure
1b), especially at elevated ![]() , is
actually due to the rapid heating of the thin layer of metal under by shoulder,
with simultaneous occurrence of a high temperature gradient by thickness of
connecting edges. To determine factors of influence that lead to a violation of
proportional nature at curves P ~ f (T)
(Figure 1c), the possibility of recrystallisation at alloy was
evaluated. For this purpose, the known relation
, is
actually due to the rapid heating of the thin layer of metal under by shoulder,
with simultaneous occurrence of a high temperature gradient by thickness of
connecting edges. To determine factors of influence that lead to a violation of
proportional nature at curves P ~ f (T)
(Figure 1c), the possibility of recrystallisation at alloy was
evaluated. For this purpose, the known relation ![]() was used, where TR and TS
- is the temperature of development static recrystallisation
and melting of metallic material, respectively. After substitution TS investigated alloy, the calculated value of TR was 100°C. Thus, under certain assumptions,
the moment of deviation from proportional of curves P ~ f (T) equal 90-95°C should
correspond to the conditions at the beginning of the development of the recrystallisation processes. In comparison with
single-phase alloys, the presence of structure particles at the second phase
can significantly change the influence of hot plastic deformation on the
development processes of primary and secondary recrystallisation.
On the other hand, the effectiveness of this effect on the grain size of the
matrix can be limited stability of existing particles second phase at hot
deformation temperatures (0.75-0.85 at FSW [8-10]. Given the presence of alloying chemical elements at alloy, the
option of forming only binary chemical compounds was considered to simplify
evaluation. According to the state diagrams of Al-Mg [11], Al-Fe [12]
and Al-Mn [13] possibility of dissolving
particles of the second phase at FSW
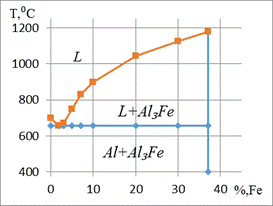
temperatures was analysed. Thus, by the Al-Mg
diagram (Figure 2a), a chemical compound Al2Mg (β-phase) must be
interacting with the matrix. For a Mg concentration
of 6.5%, the volume fraction of β-phase, considering the solution of 2.95%
of Mg atoms in a-solid solution, should be
approximately 9-9.2%. In the process of isothermal exposure, at temperatures of
approximately 375-380°C, one should expect beginning
solution Al2Mg particles
(Figure 2a). However, the small degree of overheating from the phase
equilibrium temperature indicates the need for a long enough exposure to
complete dissolution.
was used, where TR and TS
- is the temperature of development static recrystallisation
and melting of metallic material, respectively. After substitution TS investigated alloy, the calculated value of TR was 100°C. Thus, under certain assumptions,
the moment of deviation from proportional of curves P ~ f (T) equal 90-95°C should
correspond to the conditions at the beginning of the development of the recrystallisation processes. In comparison with
single-phase alloys, the presence of structure particles at the second phase
can significantly change the influence of hot plastic deformation on the
development processes of primary and secondary recrystallisation.
On the other hand, the effectiveness of this effect on the grain size of the
matrix can be limited stability of existing particles second phase at hot
deformation temperatures (0.75-0.85 at FSW [8-10]. Given the presence of alloying chemical elements at alloy, the
option of forming only binary chemical compounds was considered to simplify
evaluation. According to the state diagrams of Al-Mg [11], Al-Fe [12]
and Al-Mn [13] possibility of dissolving
particles of the second phase at FSW
temperatures was analysed. Thus, by the Al-Mg
diagram (Figure 2a), a chemical compound Al2Mg (β-phase) must be
interacting with the matrix. For a Mg concentration
of 6.5%, the volume fraction of β-phase, considering the solution of 2.95%
of Mg atoms in a-solid solution, should be
approximately 9-9.2%. In the process of isothermal exposure, at temperatures of
approximately 375-380°C, one should expect beginning
solution Al2Mg particles
(Figure 2a). However, the small degree of overheating from the phase
equilibrium temperature indicates the need for a long enough exposure to
complete dissolution.
Analysis of the state diagrams of Al-Mn [10] and Al-Fe [12] (Figure 2b) showed the presence volume fraction of
particles of Al6Mn - 3.7%, with a solution above 500°C
[13] and Al3Fe -
up to 0.6% the stably existing up to melting points of the alloy [12] (Figure
2b). At a speed of movement working tool along connecting edges of 40 mm/min,
overcoming distance of 12 mm (diameter of a shoulder) occurs in 17 s. Thus, the
total duration alloy will be under influence at weld temperatures should be
divided into effects action from the shoulder and the rod. At the beginning,
heating from a shouldered with a high temperature gradient, only one surface
each of the edges with a width of 6 mm for 8-9 s to a temperature of about 440°C.
The temperature opposite surface of the edges is lower by about 25%. Action of
the pin will be lead to additional heating (especially root of the seam) and
rapid mixing alloy on the thickness connecting edges to equalise temperature to
an average value of 380-375°C at a distance 3-4 mm from the pin.
Analysis of the state diagrams of Al-Mn
[10] and Al-Fe [12] (Figure 2b)
showed the presence volume fraction of particles of Al6Mn -
3.7%, with a solution above 500°C [13] and Al3Fe - up to 0.6% the stably existing up to melting points of the alloy [12]
(Figure 2b). At a speed of movement working tool along connecting edges of 40
mm/min, overcoming distance of 12 mm (diameter of a shoulder) occurs in 17 s.
Thus, the total duration alloy will be under influence at weld temperatures
should be divided into effects action from the shoulder and the rod. At the beginning,
heating from a shouldered with a high temperature gradient, only one surface
each of the edges with a width of 6 mm for 8-9 s to a temperature of about 440°C.
The temperature opposite surface of the edges is lower by about 25%. Action of
the pin will be lead to additional heating (especially root of the seam) and
rapid mixing alloy on the thickness connecting edges to equalise temperature to
an average value of 380-375°C at a distance 3-4 mm from the pin.
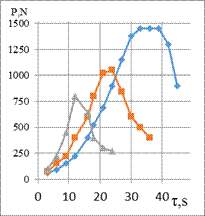
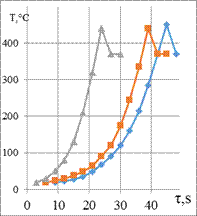
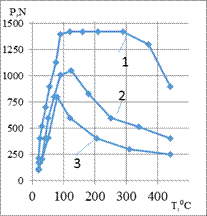
а
b
c
Fig. 1. Influence ![]() (♦ -800, ■ -1240, ▲
-1600 min
(♦ -800, ■ -1240, ▲
-1600 min![]() ) on
the change at resistance edges to immersion of the tool (a), the heating
temperature edges on the opposite side of the shoulder (b) depending duration
of the tool action and the ratio P ~ f (
T) for different
) on
the change at resistance edges to immersion of the tool (a), the heating
temperature edges on the opposite side of the shoulder (b) depending duration
of the tool action and the ratio P ~ f (
T) for different ![]()
(1-800, 2-1240, 3-1600 min![]() ) (c)
) (c)
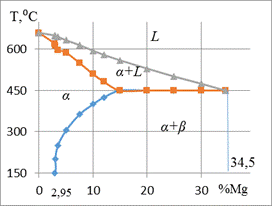
а
b
Fig. 2. Parts of state diagrams
binary alloys Al-Mg - (a) and Al-Fe - (b)
Then for the same 8-9 s, there is a further decrease at the temperature
gradient along the cross-section of the seam due to the distribution of metal
from the shoulder. It follows from the above analysis that during the period of
tool operation time, there is not enough heat for a complete dissolution of the
particles of the chemical compounds. The presence of the second phase at
structure should be considered as a factor that will help maintain a constant
grain size of the alloy matrix during FSW. Stability
of the structure during the development of secondary recrystallisation is determined by the conditions of compensation driving force of grain
boundary movement by effect of inhibition on their motion by particles of the
second phase. By these conditions, grain size (d) of alloy matrix on particle diameter second phase (D) and volume fraction (f) will be determined by the dependence
[24]:
![]() ,
(5)
,
(5)
where A is a coefficient.
For
alloys, when f > 8-10% A = 2/3 [24]. Using relation (5), it is
possible to determine the factors influencing d under the conditions of FSW development. The stability of the structure as the
alloy approaches the superplastic state
will be determined by the coalescence of the particles of the second phase,
their volume fraction and the possible solution in the matrix. In its original
state, the grain size of alloy matrix was equal to 15-17 μm. Areas with
different degrees mixing of metal edges were selected for the analysis of
structural transformations of FSW. Figure 3
shows the structures of alloy volumes in which temperature, microhardness and
grain size were measured. When forming a weld, the occurrence of a significant
difference in temperature and degree of plastic deformation near the tool rod,
lead to the formation of very complex gradient structures. In this case, there
is a significant change not only at average grain size, but also its shape.
When approaching surface near tool rod, the temperature 360°C and the
degree of plastic deformation is sufficient for formation in alloy grains of
relatively small size, with a shape close to a polygon (Figure 3d). On the
other hand, a slight increase in distance leads to the expected decrease in
temperature, for example, to 345°C (Figure 3c), and accordingly, degree of hot
plastic deformation. A characteristic feature of this area is the increased
structural heterogeneity of the formed grains. One of the reasons for the
existence of such a structure to be considered incomplete is the completion of
the recrystallisation processes [20]. For volumes of alloy
with lower temperature (Figure 3b), a structure with an even larger interval of
grain size change is observed. The location studied micro-volume relative to
the curved portion of weld (Figure 3a) indicates a more significant influence
on structure transformations from tool pin to shoulder. For average values of d (10-16 μm) and temperature range
330-360°C at f ![]() 13%, the conditional diameter particles of the second
phase was estimated by relation (5). The calculated D values were in the range of 2-3 μm, which coincides with
known data [20, 22]. Given the possible solution in particles of chemical
compounds at studied temperature range (Figure 2) in FSW, one should expect an increase at
concentration of alloying chemical elements in
13%, the conditional diameter particles of the second
phase was estimated by relation (5). The calculated D values were in the range of 2-3 μm, which coincides with
known data [20, 22]. Given the possible solution in particles of chemical
compounds at studied temperature range (Figure 2) in FSW, one should expect an increase at
concentration of alloying chemical elements in ![]() - solid
solution after cooling alloy. Then, after formation weld and its cooling, in
the layers of alloy with a high degree mixing should change the effect of
hardening from the state of solid solution. To confirm this is used the
Hall-Petch type ratio [25], with Hm as a
characteristic of strength:
- solid
solution after cooling alloy. Then, after formation weld and its cooling, in
the layers of alloy with a high degree mixing should change the effect of
hardening from the state of solid solution. To confirm this is used the
Hall-Petch type ratio [25], with Hm as a
characteristic of strength:
![]() ,
(6)
,
(6)
where Hmi is the expected microhardness of alloy matrix at
absence grain boundaries with large disorientation angles [25], ky is the angular
coefficient. Given the lack of a clear boundary in the structure between the
three zones (Figure 2b), it was decided to analyse only for two areas with
guaranteed temperatures of 330 and 360°C. The result of applying Hm against the corresponding values of d is shown in Figure 4. Execution of
this ratio indicates that level Hm is determined by the grain size of alloy matrix and
the state of the solid solution, with virtually no contribution from
substructure elements, etc. The effect on level Hm from the particles of the second phase is almost
absent, due to their location along the grain boundaries with large angles of
disorientation. The fact is that when
the particles of the second phase have a predominantly location at middle of
grains, conditions of relation (6) will be violated due to transition of the
role of the main structural element from d
to distance between the particles [20, 21, 24]. According to estimates Hm and ky, it is
determined that when temperature changes from 330 to 360°C,
contribution to the total level of microhardness from solid solution (Hm)
decreases from 400 to 110 MPa, and resistance of deformation propagation from
boundaries of grain (ky) will increase more
than twice (from 2,5 to 6 N mm-1,5). Thus, with increasing
temperature at FSW effect of
hardening from the state of solid solution decreases, while the grain
boundaries increases. Comparative analysis Hm for the
equal d but at different temperatures
at FSW shows a violation of the known ratio, when
under normal conditions of deformation decrease temperature increases the
hardening effect, and reduces the degree of softening alloy. Thus, for d = 8 μm, at 330°C, Hm is less
than 700 MPa, and for 360°C on 15% higher (![]() ). For a big d, different relationship is obtained. For example, at d = 28 μm, Hm = 520 MPa
for a temperature of 330°C, and for 360°C, Hm = 480 MPa
(
). For a big d, different relationship is obtained. For example, at d = 28 μm, Hm = 520 MPa
for a temperature of 330°C, and for 360°C, Hm = 480 MPa
(![]() ). Given that with increasing d, the parameters of strain hardening
increase [21], to the known requirements for achieving over superplastic flow, in addition to
maintaining a stable structure with over fine grain, it should further minimise
influence from the state of solid solution.
). Given that with increasing d, the parameters of strain hardening
increase [21], to the known requirements for achieving over superplastic flow, in addition to
maintaining a stable structure with over fine grain, it should further minimise
influence from the state of solid solution.
5. CONCLUSIONS
1.
At temperatures
above phase equilibrium, the partial solution of particles chemical compounds
is a source of supersaturation solid solution by atoms alloying elements under
FSW.
2.
At the formation
of weld, the presence in structure particles of the second phase is a factor in
inhibiting movement grain boundaries at the period of structure formation.
3.
Increasing
temperature during FSW, accompany by decrease contribution from state of solid
solution and increased role of grain boundaries to the overall hardness level
of the weld.
Gratitude
The authors are grateful to the Chief of the
laboratory, Yu. L. Nadezhdin, for his valuable advice on discussing research
results.


а
b


c
d
Fig. 3. General view of the area intensive mixing after FSW (![]() = 1250 min
= 1250 min![]() ) (a)
) (a)
and enlarged image of the selected fragment depending on heating temperature:
(b) -330,
(c) -345 and (d) -360°C. Magnification: a - 100; b-d - 350
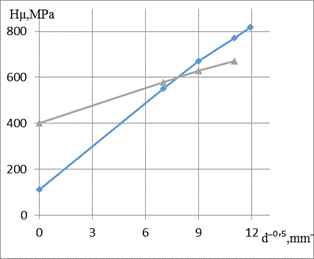
Fig. 4. Influence of the size of grain ![]() - solid
solution on microhardness,
- solid
solution on microhardness,
depending on the temperature of the microvolumes alloy at FSW (▲ - 330,
♦ -360°C)
References
1.
Ding J., R. Carter, K. Lawless, et.al. 2006. „Friction stir
welding flies high at NASA”. Ibid 3: 54-59.
2.
Hovanski Yu., P. Upadhyay, J. Carsley, et.al. 2015. „High-Speed
Friction-Stir Welding to Enable Aluminum Tailor-Welded Blanks”. The
Journal of The Minerals, Metals & Materials Society 67: 1045-1053. ISSN:
1047-4838. DOI: https://doi.org/10.1007/s11837-015-1384-x.
3.
MST Technology Co., LTD. 2020. „Friction Stir Welding Applied To
Rail”. Available at:
https://www.aee-fsw.com/news/friction-stir-welding-applied-to-rail-33051203.html.
4.
Bušić
M., Z. Kožuh, D. Klobčar, I. Samardžić. 2016.
“Friction stir welding (FSW) of aluminium foam sandwich panels”. Metalurgija 55(3): 473-476.
5.
Miličić
M., P. Gladović, R. Bojanić, T. Savković, N. Stojić. 2016.
“Friction stir welding (FSW) process of copper alloys”. Metalurgija 55(1): 107-110.
6.
Podržaj P.,
B. Jerman, D. Klobčar. 2015. “Welding defects at friction stir
welding”. Metalurgija 54(2):
387-389.
7.
Klobčar D.,
J. Tušek, M. Bizjak, V. Lešer. 2014. “Micro friction stir
welding of copper electrical contacts”. Metalurgija 53(4): 509-512.
8.
Thomas W.M., E.D.
Nicholas, J.C. Needham, et. al. Patent 5,460,317. United States. Friction welding. Assignee The
Welding Institute Cambridge, United Kingdom. Public date: 10.10.1995. Available at:
https://patents.google.com/patent/US5460317A/en.
9.
Yihua Xiao, Haifei
Zhan, Yuantong Gu, et.al. 2017. “Modeling heat transfer during friction
stir welding using a meshless particle method”. International Journal of Heat and Mass Transfer 104: 288-300. DOI:
10.1016/j.ijheatmasstransfer.2016.08.047.
10.
Dalder E., J.W.
Pastrnak, J. Engel, et.al. 2008. “Friction stir welding of thick-walled
aluminum pressure vessels”. P. 40-44. Available at:
https://app.aws.org/www/wj/2008/04/WJ_2008_04.pdf.
11.
Mola K., A. Dziadon.
2008. “Formation of magnesium – eutectic mixture layered
composite”. Archives of Foundry
Engineering 8: 127-132.
12.
Li X., A. Schert,
M. Heilmaier, et. al. 2016. “The
Al – rich Part of the Fe – Al Phase Diagram”. Journal of Phase
Equilibria and Diffusion 37: 162-173.
13.
Kematick R.J.,
C.E. Myers. 1992. “Thermodynamics and Phase Equilibria in the Al-Mn
System”. Journal of Alloys and Compounds 178: 343-349.
14.
Villegas J.F., J.V. Dominguez,
G.V. Ochoa, et.al. 2017. “Thermo-mechanical
modeling of friction-stir welding tool used in aluminum alloys joints”. Contemporary Engineering Sciences 10(34):
1659-1667. DOI: 10.12988/ces.2017.711156.
15.
Li Dongxiao, Yang
Xinqi, Cui Lei, et.al. 2015. “Investigation of stationary shoulder
friction stir welding of aluminum alloy 7075-T651”. Journal of Materials Processing Technology 222: 391-398. DOI:
10.1016/j.jmatprotec.2015.03.036.
16.
El-Sayed M.M, A.Y.
Shash, T.S. Mahmoud, et. al. 2018. “Effect of friction stir welding
parameters on the peak temperature and the mechanical properties of aluminum
alloy 5083-O”. Improved Performance
of Materials 72: 11-25.
DOI: 10.1007/978-3-319-59590-0_2.
17.
Dawes C.J. 1995.
“An introduction to friction stir welding and its development”. Weld. and metal fabr. 1: 13-16.
18.
Wilkinson D.S., C.H. Caceres.
1984. “On the mechanism of strain-enhanced grain grown
during super plastic
deformation”. Acta Met. 32:
1335-1345.
19. Partridge P.G., D.S. Mc Darmaid, A.W. Bowen. 1985.
“A deformation model for anisotropic super plasticity in to phase alloys”. Acta Met. 33: 571-577.
20.
Xiao-guo Wang, Qiu-shu Li, Rui-rui Wu, et al. 2018. “A Review on Superplastic Formation Behavior
of Al Alloys”. Advances in
Materials Science and Engineering 7606140. DOI:
https://doi.org/10.1155/2018/7606140.
21.
Ruano O., O.D. Sherby.
1988. “On constitutive equations for various diffusion-controlled creep
mechanisms”. Revue de Physique Appliquee 23:
625-637.
22.
Sakai G., Z.
Horita, T.G. Langdon. 2005. “Grain refinement and superplasticity in an
aluminum alloy processed by high-pressure torsion”. Materials Science
and Engineering A 393: 344-351.
23.
Robiller G., Ch. Straβburger. 1969. “Zum Bauchinger–effektunlergiester stahle”. Materialprufung 1: 89-95.
24.
Smith C.S. 1948. “Grains, phases and interfaces: an interpretation
of microstructure”. Trans. ASME
175: 15-67.
25.
Christ B.W., G.V. Smith.
1967. “Comparison of the hall-petch parameters of zone-refined iron
determined by the grain size and extrapolation methods”. Acta Metallurgica 15: 809-816. DOI:
https://doi.org/10.1016/0001-6160(67)90362-8.
Received 07.04.2021; accepted in revised form 24.05.2021
![]()
Scientific
Journal of Silesian University of Technology. Series Transport is licensed
under a Creative Commons Attribution 4.0 International License