Article citation information:
Dźwigoń, W. Analysis of transition times of
pedestrians and passengers in an interchange node. Scientific Journal of Silesian University of Technology. Series
Transport. 2016, 92,
31-40. ISSN: 0209-3324.
DOI: 10.20858/sjsutst.2016.92.4.
Wiesław DŹWIGOŃ[1]
ANALYSIS OF TRANSITION TIMES
OF PEDESTRIANS AND PASSENGERS IN AN INTERCHANGE NODE
Summary.
Accurate design of infrastructure for public transport is the basis for the
efficient functioning of traffic and passenger transportation. The article
presents an analysis of the availability of public transport stops. The measure
of the availability relates to access times to certain stops from other stops
and the surroundings of the transport hub. The article discusses the scope and the objective
of measuring pedestrian and passenger traffic. It also presents an analysis
of transition times for the passengers who change their means of transport and
need to reach a stop. The provided measurements were carried out on a two-level
interchange tram-bus hub. Thus they should be part of any assessment of the
quality of passenger service in the hub.
Keywords:
public transport; interchange node; transit time.
1. INTRODUCTION
Change is an important component of
the journey undertaken by means of public transport. Some of the changes are
performed in specially designed interchange nodes [1, 5]. They can have a
considerable degree of complexity in terms of their infrastructure.
Additionally, stops located at the interchange nodes
offer services to passengers from the surrounding areas who wish to use public
transport vehicles [4].
There are a few methods that focus
on the evaluation of interchange nodes [3, 6]. However, most often, a change is
one of the components of a qualitative assessment of passenger service, be it a
partial or a synthetic evaluation [7, 8]. The partial criteria present in such
an assessment include:
· the
time lost when changing the means of transport (transition between stops,
waiting for the vehicle to arrive)
· the
distance between stops
· the
conditions when accessing stops (possibility of collision, ease of orientation,
traffic lights, height differences, density of pedestrian traffic)
· the
conditions when waiting at the bus stop (shelter, attractive surroundings, density of
pedestrian traffic).
The paper focuses on the analysis of
the transit times between stops localized at the interchange node.
Measurements included the entire area of the interchange node, which also
allowed for capturing the connections between the node and the surroundings, but only in the area of the
interchange node. The results ought to be used to evaluate solutions that are
formulated from the point of view of the passenger and pedestrian traffic.
2. CONDUCTING MEASUREMENTS
The aim of the measurements was to
assess the usefulness of the method being used, as well as obtain data for
the partial assessment of the functioning of the interchange. In this case, the
transition time is a component of a fractional assessment.
For the analysis, a characteristic
traffic hub was selected, namely, the Mogilskie
roundabout in Kraków. It is situated on the border of
the city’s downtown area. Cross-town and tangential bus and tram lines run
through it. It is also part of the second ring road, which generates intense
traffic for the majority of connection types. The most important idea
accompanying the reconstruction was to separate vehicle traffic from pedestrian
traffic and public transport vehicles. The aim in doing so was to increase the
efficiency of public transport and the improvement of safety. Finally, the
completion of a two-level road junction was executed with the following
specifications:
· there
is traffic comprising cars and buses on the ground level (level 0)
· there
is traffic comprising trams, pedestrians and cyclists on the lower level (level
-1)
Figure 1 shows a diagram of this
node. Five streets feed into the intersection (including four dual
carriageways), while there is a bus stop at each exit from the roundabout. On the lower
level, there are tram tracks, three of which lead to the street of the upper
level and one to a tunnel. All pedestrian and bicycle traffic is performed on
the lower level. This implies the need to overcome differences in height
between the trams and bus stops, as well as between tram stops and the surroundings of the node (using ordinary stairs,
escalators [2], ramps or lifts). Meanwhile, pedestrians passing among the
surrounding buildings and passing through the node must be twice the difference
in height between the levels. On the lower level next to the tram stops, there
are three pedestrian crossings with traffic lights. Most pedestrians and
interchange users have to use them, which could extend the transition times and
times for any interchange. In addition, the trams passing through the lower
level lose time due to waiting on these traffic lights. Bicycle traffic on the
lower level generates a problem of having to make up the differences in terms
of twice the height. The lack of collisions with car traffic is an advantage of
this solution.
The lower node level is not
symmetrical; it is far from the tram stops to the others stops and those
buildings that are located on the northern side of the node. On the other hand,
it is close to the southern direction. Many office buildings surrounding the
node generate significant pedestrian traffic, which is directed to the stops.
Additionally, they produce large flows of passengers who switch their means of
transport when selecting connection options. Summing up, on the lower level, a
surface with a large degree of pedestrian and cycling traffic was created,
where everyone enters from different directions.
The specific objectives of
measurement include:
· determining
transition times in different types of connections in the node (among stops and
between the stops and the surroundings)
· determining
time losses for these types of connections
· determining
the reasons for time losses (reasons for stoppages)
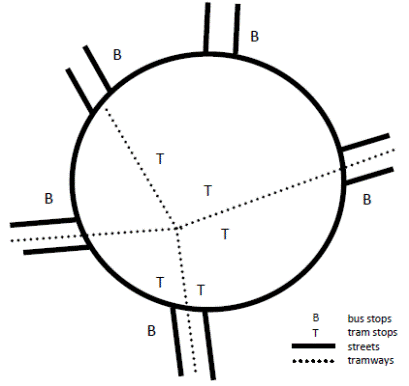
Fig. 1. Diagram of the analysed transport
node
The survey was performed using a
method of tracking a pedestrian or a transport passenger who appeared at the
interchange node, came from the surrounding area or got off at a bus stop. The
person taking the measurements recorded a variety of pedestrian behaviours.
After one pedestrian left the node, the person taking the measurements randomly
chose another person whose behaviour was to be measured. This kind of
measurement method means that large flows of passengers (or pedestrians) can be
measured many times, resulting in a large sample being obtained. Conversely,
where small flows are involved, the sample will be small and unreliable. The
analysis only included the area of the node (up to its borders) and was not
related to any further connections between the node and its surroundings.
In the process of taking
measurements, the following types of information were recorded:
· the
places where a pedestrian appeared and left the node (stops of quarters
building)
· the
transition time between these points
· the
places and reasons for stoppages (traffic lights, traffic conflict, small
purchases, other)
· the
time of stoppages
· the
ways to overcome differences in height (ordinary stairs, escalators, ramps,
elevator)
Finally, the actions of 531
individuals were measured and the structure of the measured dependencies is as
follows:
· the
movement of passengers between stops = 59%
· the
movement of passengers between stops and the surroundings of the interchange node = 32%
· pedestrian
traffic passing through the node = 9%
3. ANALYSIS OF THE RESULTS
The analysis firstly clustered the
types of connections into several groups (for example, stop-stop or stop-surroundings), followed by types of connections
between the individual stops. Those types of connections when only several measurements
were obtained were skipped (between bus stops and from bus stops to the surroundings of the node). Figure 2 summarizes
the average transition times. It is noteworthy that the shortest time between
tram stops is 82 s. This is related to their location being on the lower level
of the node and the short distance between them. All other types of connections
clearly have longer transition times. This is related to three factors:
· the
greater distance to a crossing
· the
difference in height between the levels
· the
necessity to go through pedestrian crossings, where some people lose time when
waiting on traffic lights
The types of connections involving
“tram stop-surroundings” require half the diameter of a node to be made, as well as the
same difference in height, while the types of connections involving “bus-bus”
and “surroundings-surroundings” require a full diameter of the node and twice the height difference.
Table 1 lists more statistical parameters describing the analysed transition
times: sample size, average, standard deviation, variation coefficient, and
percentiles p5 and p95.
Generally, transition times are characterized by high volatility. The variation
coefficient ranges from 0.39 to 0.59 for different groups of connection types.
This is connected with different distances between the stops within one group.
What is also significant is the presence of traffic lights at pedestrian
crossings. For example, in the types of connections between different tram
stops, there are zero, one or two pedestrian crossings. This means that a
passenger, while changing the means of transport, may lose between 0 and 80 s
due to traffic lights, with an average walking time equal to 82 s. The
consequence of this is high volatility in the transition time. In addition, the
speed of passengers along the access passages to the stops is very volatile.
When a passenger sees a tram at the stop, they speed up in order to catch the
tram. Conversely, when seeing a stop with no vehicle, a passenger slows down,
because there is no reason to hurry.
Tests of significance for two
averages, carried out at a confidence level of 0.95, showed that:
· transition
times in “tram stop-bus stop” and in the opposite direction do not differ
significantly; while
· transition times
in “tram stop-surroundings” and in the
opposite direction do not differ either.
Therefore, they will be grouped in
the subsequent analysis. For types of connections grouped in this way, the
transition times were estimated. Table 2 summarizes the limits of confidence
intervals.
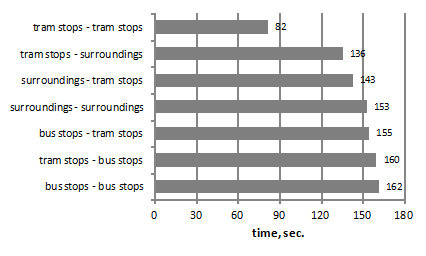
Fig. 2. Average transition times through
the node for different groups (in s)
Table 1. Characteristics
of transition times for different types of connection groups
|
Connection |
Count |
Average |
Standard deviation |
Variation coefficient |
Percentile p5 |
Percentile p95 |
|
Tram
stops-bus stops |
36 |
160 |
68 |
0.42 |
77 |
263 |
|
Bus
stops-tram stops |
40 |
155 |
60 |
0.39 |
67 |
268 |
|
Bus
stops-bus stops |
12 |
162 |
69 |
0.43 |
90 |
275 |
|
Tram
stops-tram stops |
224 |
82 |
40 |
0.49 |
30 |
155 |
|
Tram
stops-surroundings |
104 |
136 |
61 |
0.45 |
54 |
273 |
|
Surroundings-tram
stops |
61 |
143 |
84 |
0.59 |
37 |
292 |
|
Surroundings-surroundings |
45 |
153 |
66 |
0.43 |
66 |
254 |
The error in estimating the
transition time for various types of connections is as follows:
· for the
passage of pedestrians through a node = 12.5%
· between
tram stops and the surroundings = 7.9%
· between
tram stops and bus stops = 8.5%
· between
tram stops = 6.4%
· between
all the stops = 6.1%
Clearly, the error estimate
decreases with an increase of the size of the measured sample.
Table 2. Characteristics
of confidence intervals for different types of connections groups
|
Connection |
Count |
Lower limit |
Average |
Upper limit |
|
Surroundings-surroundings |
45 |
134 |
153 |
172 |
|
Tram
stops-surroundings |
163 |
126 |
137 |
148 |
|
Tram
stops-bus stops |
76 |
140 |
153 |
166 |
|
Tram
stops-tram stops |
224 |
77 |
82 |
87 |
|
All stops |
312 |
96 |
102 |
108 |
The graphs in Figures 3, 4 and 5
show the distribution of transition times for various types of connections.
Clearly, the graph for the
transition times between the tram and bus stops is most concentrated (the graph
in Figure 3 is almost symmetrical), with the coefficient of variation only
being 0.38. In two other cases, it amounts to 0.49 and 0.51. In Figures 4 and
5, approximately 10% of the measurements represent elongated transition times,
which is mainly caused by the crossings with traffic lights.
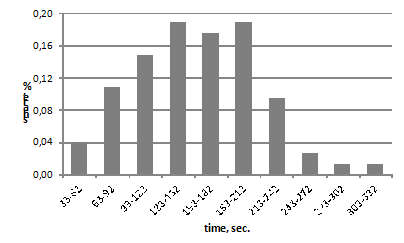
Fig. 3. Transition time between tram and
bus stops
The graph in Figure 6 shows the time
distribution functions of passage. The average transition time for changes
between the closest situated tram stops is 82 s. Contemplating changes between
tram-bus and bus-bus elongates the average transition time by up to 102 s.
Distances to bus stops are bigger and there is a need to overcome the
differences in height, which increases the transition times. Transition times
are elongated by approximately 13%, starting from the p80
percentile.
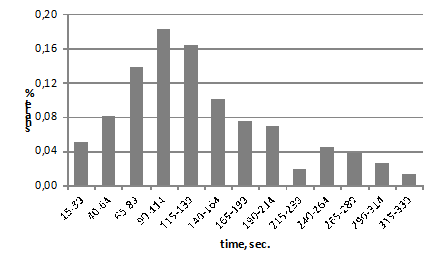
Fig. 4. Transition time between tram and
bus stops
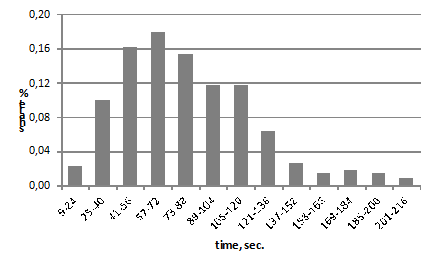
Fig. 5. Transition time between tram and
bus stops
The final stage of the analysis is
to determine the effect of the stoppages in relation to transition times. The
structure of the measured passages is as follows:
·
passages without detentions = 63.9%
·
passages with one detention = 32.8%
·
passages with two detentions = 4.3%
The average passage without
detention lasts 103 s, although one detention extends it to 142 s and two
detentions extends it by up to 244 s (which is more than 100% more). Table 3
summarizes the transition times for each types of connections (a very small
sample size with less than seven measurements was omitted).
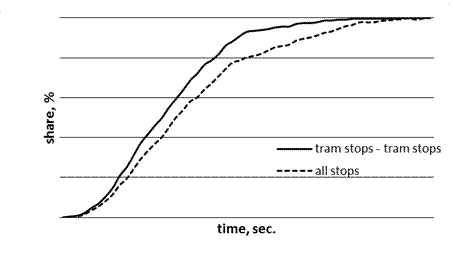
Fig. 6. Transition time of distribution
functions between the tram stops (continuous line) and between all stops of the
node (dotted line)
Table 3. Characteristics
of transition times for certain types of connections in the node
|
Connection |
Count |
Average |
Standard deviation |
Variation coefficient |
Pedestrian crossings |
||||||
|
Surroundings-surroundings |
|||||||||||
|
S1-S2 |
15 |
115 |
59 |
0.52 |
0 |
||||||
|
S2-S3 |
11 |
163 |
44 |
0.27 |
1 |
||||||
|
S2-S5 |
9 |
168 |
56 |
0.33 |
1 |
||||||
|
tram stops-bus stops |
|||||||||||
|
T1-B2 |
7 |
128 |
30 |
0.23 |
0 |
||||||
|
T2-B2 |
7 |
135 |
24 |
0.18 |
0 |
||||||
|
T3-B2 |
15 |
170 |
50 |
0.29 |
1 |
||||||
|
T4-B2 |
7 |
150 |
75 |
0.50 |
1 |
||||||
|
T5-B2 |
7 |
219 |
48 |
0.22 |
2 |
||||||
|
Tram stops-surroundings |
|||||||||||
|
S2-T1 |
18 |
120 |
54 |
0.44 |
0 |
||||||
|
S2-T2 |
15 |
128 |
68 |
0.53 |
0 |
||||||
|
S3-T3 |
13 |
85 |
35 |
0.41 |
0 |
||||||
|
S2-T3 |
35 |
144 |
57 |
0.40 |
1 |
||||||
|
S2-T5 |
19 |
225 |
69 |
0.31 |
2 |
||||||
|
Tram stops-tram stops |
|
||||||||||
|
T1-T2 |
44 |
65 |
42 |
0.65 |
0 |
|
|||||
|
T1-T3 |
58 |
68 |
29 |
0.42 |
1 |
|
|||||
|
T1-T4 |
15 |
96 |
32 |
0.33 |
1 |
|
|||||
|
T3-T5 |
46 |
97 |
41 |
0.43 |
1 |
|
|||||
|
T1-T5 |
16 |
123 |
48 |
0.39 |
2 |
|
|||||
|
T2-T5 |
21 |
98 |
29 |
0.30 |
2 |
|
|||||
T = tram stop; B =
bus stop; S = surroundings
In each group of the type of
connection (surroundings-surroundings, tram-tram etc.), it is evident that the
shortest transition times are related to the types of connections, meaning that
they are not vulnerable to any loss of time regarding red traffic lights. The
need to pass through traffic lights, especially twice, clearly increases the
transition time. This applies particularly to the types of connections between
the lower and upper levels of the interchange node, meaning changes between a
bus and a tram as well as walks from the surroundings of the hub to tram stops. The least
accessible place is the T5 tram stop, which has the
longest lead times between it and the surroundings, as well as from bus and tram stops. For many
passengers, access to this stop requires overcoming two pedestrian crossings,
each of which have traffic lights.
5. SUMMARY
The analysis enables the evaluation
of the usefulness of the respective method of measurement in relation to the
characteristics of pedestrian and passenger interchange behaviour. The method
of tracking and manually recording behaviours of pedestrians is very accurate,
as it allows for identifying the durations of different situations with an
accuracy up to one second. The measurement form can be easily modified, for
example, the one-level node where people cross at the red or green light can be
recorded (instead of a method for overcoming the differences in height, which
do not occur in this case).
Transition times are affected by the
distance between the stops and the need to go through pedestrian crossings with
traffic lights. In the case of the analysed infrastructure, the average
transition time without stopping is 103 s. One stop increases it by 40%, while
two stops increases it by more than 100%. Transition times are characterized by
high volatility, which is associated with the behaviour of a pedestrian with
regard to acceleration or deceleration, depending on the situation at the
target stop, talking on a mobile phone etc. Such situations were not recorded.
The results may be useful when:
· performing
a simulation analysis of the functioning of an interchange node, for example,
using VISSIM software
· evaluating
the interchange node
· designing
other interchange nodes
· assessing the
quality of a journey made by public transport.
References
1.
Duda Urszula. 2015. Planowanie i projektowanie
stref krótkiego parkowania (kiss and ride) jako
elementu efektywnego węzła przesiadkowego. In Współczesny stan wiedzy w inżynierii lądowej, edited by Joanna Bzówka, 119-129.
Gliwice: Wydawnictwo Politechniki Śląskiej. ISBN 978-83-7880-207-5. [In Polish: Planning and
designing a short-term park zone (kiss and ride) as an element of an
effective transport node. In The Modern
State of Knowledge in Civil Engineering. Gliwice: Gliwice: Silesian
University of Technology Publishing].
2.
Dźwigoń Wiesław. 2014.
“Efficiency of escalators in public transport”. Logistics 4: 2781-2789. ISSN 1231-5478.
3.
Dźwigoń Wiesław. 2012. “Kryterium oceny bezpieczeństwa w ocenie węzłów
przesiadkowych”. Transport Miejski i Regionalny 6: 13-18. ISSN 1732-5153.
[In Polish: “Criterion for the safety of passengers in the evaluation of
interchange nodes”. Urban and Regional
Transport 6: 13-18].
4.
Gaca Stanisław, Wojciech Suchorzewski,
Marian Tracz. 2008. Inżynieria ruchu
drogowego. Teoria i praktyka. Warsaw: Wydawnictwa
Komunikacji i Łączności. ISBN 978-83-206-1707-8. [In Polish:
Road traffic
engineering. Theory and practice. Warsaw: Transport and
Communication Publishers].
5.
Husarek Piotr, Wojciech Palus. 2005. “Analiza funkcjonowania węzłów
przesiadkowych w transporcie zbiorowym”. Transport Miejski i Regionalny 11: 2-8. ISSN 1732-5153.
[In Polish: “Functional analysis of interchange nodes in public transport”. Urban and Regional Transport 11: 2-8.
ISSN 1732-5153].
6.
Olszewski Piotr, Halina Krukowska, Piotr Krukowski.
2014. “Wskaźnikowa metoda oceny węzłów przesiadkowych transportu publicznego”. Transport Miejski i Regionalny 6: 4-9. ISSN 1732-5153. [In Polish: “Indicator method for public
transport interchange node evaluation”. Urban
and Regional Transport 6: 4-9].
7.
Rudnicki Andrzej. 1999. Jakość komunikacji miejskiej. Cracow:
Stowarzyszenie Inżynierów i Techników Komunikacji. ISSN 1231-9155. [In Polish: Urban public transport quality. Cracow:
Polish Association of Transport Engineers and Technicians].
8.
Starowicz Wiesław. 2007. Jakość przewozów w miejskim transporcie zbiorowym. Cracow: Wydawnictwo Politechniki Krakowskiej. ISBN
978-83-7242-427-3. [In Polish: Quality of urban public
transport. Cracow:
Cracow University of Technology Publishing].
Received 11.04.2016;
accepted in revised form 25.07.2016
![]()
Scientific Journal of Silesian University of
Technology. Series Transport is licensed under a Creative Commons Attribution
4.0 International License